Understanding Elementary Shapes

1. LINES AND ANGLES
Babilonians used geometry during the period 3000 BC to 2000 BC. They found formulae for areas and perimeters of rectangle and square. To mark the boundaries of the fields that were affected by the floods due to the over flow of river Nile and to compute their areas, geometry came to help of Egyptians. From ancient Vedic times geometry got special importance in our country. Geometry was developed as a science along with astronomy in our country. ‘Kalpa’ is a part of vedas. Later on, geometry was developed as ‘sulbha sutras’. Great people like Boudhayana, Apasthambha, Katyana etc., became famous authors of such ‘sulbha sutras’.
Basic Geometrical Concepts
Axioms:  The basic facts which are taken for granted without proof are called axioms.
Eg:
• Halves of equals are equal
• The whole is greater than each of its parts
• A line contains infinitely many points
Statements: A sentence which can be judged to be true or false is called a statement.
Eg:
• The sum of the angles of a triangle is 1800 is a true statement
• x + 8 > 12 is a sentence but not a statement
Theorems: A statement that requires a proof is called a theorem. Establishing the truth of a theorem is known as proving the theorem.
Eg:
The sum of the angles of a triangle is 180°.
Corollary: A statement, whose truth can easily be deducted from a theorem, is called its corollary.
Fundamental Geometrical Terms
Point: A point is a mark of position. A fine dot represents a point. We denote a point by a capital letter A, B, P, Q etc. A point has no length, breadth or thickness.
Line Segment: The straight path between two points A and B is the line segment AB, represented as $\overline{\mathrm{AB}}$ .
The points A and B are called its end points.
A line segment has a definite length.

The distance between two points A and B gives the length of the line segment $\overline{\mathrm{AB}}$ .
Ray: A line segment AB when extended indefinitely in one direction is the ray $\overrightarrow{\mathrm{AB}}$ .
It has one end point A.

A ray has no definite length. A ray cannot be drawn, it can simply be represented on the plane of a paper.

Line: A line segment AB when extended indefinitely in both the directions is called line $\overleftrightarrow{\mathrm{AB}}$ .

A line has no end points. A line has no definite length. A line cannot be drawn, it can simply be represented on the plane of a paper.
Incidence Axioms on Lines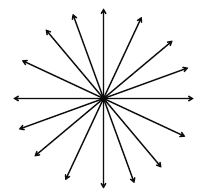

• A line contains infinitely many points
• An infinite number of lines can be drawn to pass through a given point
• One and only one line can be drawn to pass through two given points A and B.

Collinear Points: Three or more points are said to be collinear, if there is a line which contains them all.

In the above figure; P, Q, R are collinear points.
Plane: A flat surface extended endlessly in all the four directions is called a plane. The surface of a smooth wall, the surface of the top of the table, the surface of a smooth blackboard, the surface of a sheet of paper etc. are close examples of a plane. These surfaces are limited in extent but the geometrical plane extends endlessly in all directions. Two lines lying in a plane either intersect exactly at one point or are parallel.
Intersecting Lines: Two lines having a common point are called intersecting lines. The point common to two given lines is called their point of intersection. In the figure, the lines AB and CD intersect at a point O.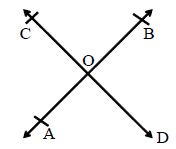

Concurrent Lines: Three or more lines in a plane are said to be concurrent, if all of them intersect at the same point. In the figure below, the lines l, m, n all intersect at the same point P; so they are concurrent.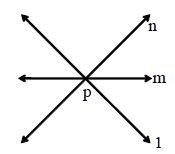

Parallel Lines: Two lines l and m in a plane are said to be parallel, if they have no point in common and is written as l || m. The distance between two parallel lines always remains the same.

Angles: Two rays OA and OB having a common end point O form angle AOB, written as $\ang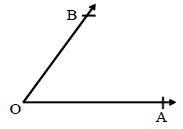le$AOB.

OA and OB are called the arms of the angle and O is called as its vertex.
Measure of an Angle: The amount of turning from OA to OB is called the measure of $\angle$AOB is written as $\angle$ AOB. An angle is measured in degrees, denoted by.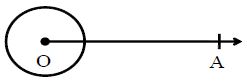

A complete rotation around a point makes an angle of 360°.
1° = 60 minutes, written as 60′ .
1′ = 60 seconds, written as 60″ .
We use a protractor to measure an angle.
Kinds of Angles
• Right Angle: An angle whose measure is 90° is called a right angle.
• Acute Angle: An angle whose measure is more than 0° but less than 90°, is called an angle.
• Obtuse Angle: An angle whose measure is more than 90° but less than 180° is called an obtuse angle.
• Straight Angle: An angle whose measure is 180° is called a straight angle.
• Reflex Angle: An angle whose measure is more than 180° but less than 360° is called a reflex angle.
• Complete Angle: An angle whose measure is 360° is called a complete Angle.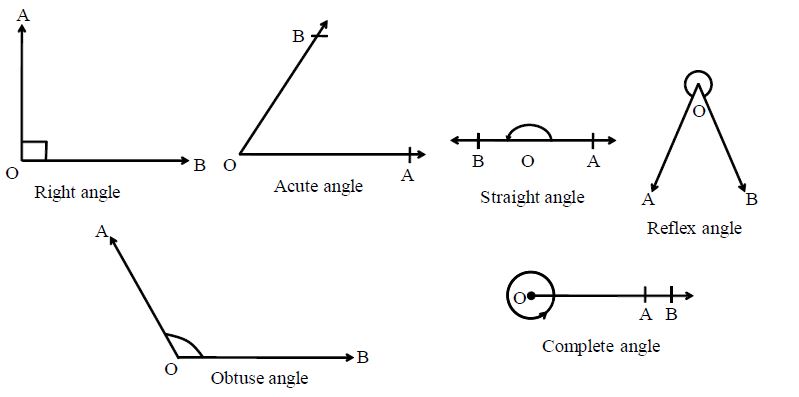

• Equal Angles: Two angles are said to be equal, if they have the same measure.
• Bisector of an Angle: A ray OC is called the bisector of $\angle$AOB, if m $\angle$AOC= m $\angle$BOC.

• Complementary Angles: Two angles are said to be complementary, if the sum of their measures is 90°.
Two complementary angles are called the complement of each other.
Thus, Complement of an angle of 36° = An angle of (90° – 36°) = 54°.
• Supplementary Angles: Two angles are said to be supplementary, if the sum of their measures is 180°. Two supplementary angles are called the supplement of each other.
Thus, supplement of an angle of 42° = An angle of (180° – 42°) = 138°.
Supplement of an angle of 115° = an angle of (180° – 115°) = 65°.
• Adjacent Angles: Two angles are said to be adjacent angles, if they have a common vertex and a common arm such that the other arms of the two angles are on either side of their common arm.
In the adjoining figure, $\angle$AOB and $\angle$BOC are adjacent angles.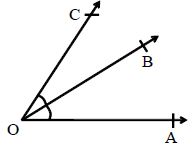

Linear Pair: If the sum of two adjacent angles is 180°, they are said to form a linear pair. In the adjoining figure, $\angle$AOB + $\angle$COB = .
So AOB and BOC together form a linear pair.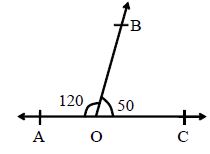

Important Results
Linear Pair Axiom: If a ray stands on a straight line, then the sum of the adjacent angles so formed is 180°. Thus, $\angle$AOC + $\angle$COB = 180° where AOB is a straight line.

Converse of a Linear Pair Axiom: If two adjacent angles are supplementary, then the non-common arms of two angles are in a straight line. Thus if two adjacent angles $\angle$AOC and $\angle$BOC with common arm OB are such that $\angle$ AOC + $\angle$BOC = 180° then OA and OB are in the same straight line i.e. AOB is a straight line.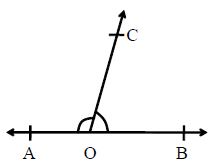

Angles at a Point: The sum of all the angles at a point is 360°.
In the given figure, we have ∠1 + ∠2 + ∠3 + ∠4 = 360°.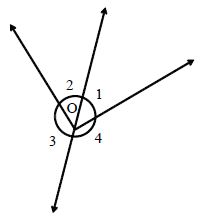

Vertically Opposite Angles: If two straight lines AB and CD intersect at a point O, then ∠AOC and ∠BOD form one pair of vertically opposite angles and the angles AOD and BOC form another pair of vertically opposite angles. When two lines intersect each other, then vertically opposite angles are always equal. In the given figure, we have: ∠AOC = ∠BOD and ∠BOC = ∠AOD.

Parallel Lines: Two straight lines lying in the same plane are said to be parallel if they do not intersect no matter how long they are produced on either side. In the given figure, AB and CD are parallel lines and we write AB || CD.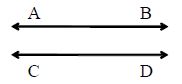

Transversal: A straight line that intersects two or more lines is called a transversal. Let two lines AB and CD are cut by a transversal l, then the following angles are formed.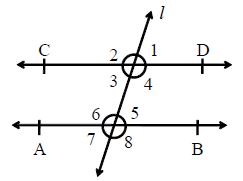

• Pairs of Corresponding Angles (abbreviated as corres ∠s): (∠1, ∠5), (∠2, ∠6), (∠4, ∠8), (∠3, ∠7)
• Pairs of Alternate Interior Angles (abbreviated as Alt. Int. ∠s): (∠3, ∠5) and (∠4, ∠6)
• Pairs of Alternate Exterior Angles: (∠2, ∠8) and (∠1, ∠7)
• Pairs of Consecutive Interior Angles (abbreviated as co. Int. s): (∠4, ∠5) and (∠3, ∠6)
Properties of Angles Associated with Parallel Lines
If two parallel straight lines are intersected by a transversal, then
• Corresponding angles are equal. ∠1 = ∠5, ∠2 = ∠6, ∠4 = ∠8 and ∠3 = ∠7
• Alternate interior angles are equal. ∠3 = ∠5 and ∠4 = ∠6
• Alternate exterior angles are equal. ∠2 = ∠8 and ∠1 = ∠7
• Co-interior angles (consecutive interiors) are supplementary. ∠4 + ∠5 = 180° and ∠3 + ∠6 =180°.
Conditions of Parallelism: The converse of the above results is also true.
• If two straight lines are intersected by transversal such that a pair of corresponding angles are equal, then the two lines are parallel.
• If two straight lines are intersected by a transversal such that a pair of alternate angles are equal, then the two lines are parallel.
• If two straight lines are intersected by a transversal such that a pair of consecutive interior angles is supplementary, then the two lines are parallel.
Basic Axiom of Parallel Lines: (Euclidian postulate)
• There exists one and only one line which is parallel to a given line from a given point.
• If two lines are parallel to the same line then the lines are parallel to each other.

2. TRIANGLE
A closed plane figure bounded by three line segments is called a Triangle. We denote a triangle by the symbol $∆$. $∆$ABC has:
• Three vertices, namely A, B and C
• Three sides, namely AB, BC and CA
• Three angles, namely, ∠A, ∠B and ∠C.

The three sides and the three angles are known as elements or parts of the Triangle.
Kinds of Triangles
Classification of Triangles according to Sides:
Scalene Triangle: A triangle, in which all sides are of different lengths, is called a scalene triangle.
In the following figure, $∆\mathrm{PQR}$ is a scalene triangle

since $\mathrm{PQ}\ne \mathrm{QR}\ne \mathrm{PR}$
Isosceles Triangle: In an isosceles triangle, the angles opposite to the equal sides are equal. A triangle having two sides equal is called an isosceles triangle.

In $\Delta \mathrm{ABC}; \mathrm{AB}=\mathrm{AC}\Rightarrow \angle \mathrm{B}=\angle \mathrm{C}$.
Equilateral Triangle: A triangle which is having equal sides is called an equilateral triangle. The measure of each angle of an equilateral triangle is 60°.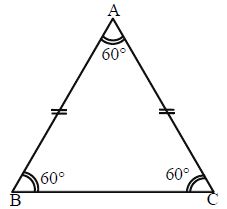

Classification of Triangles according to Angles:
Acute-Angled Triangle: A triangle, in which every angle measures more than 0° but less than 90° is called an acute-angled triangle. In the below figure $∆$PQR, we have: ∠P = 70°, Q = 45° and R = 65°.
Thus, each angle of PQR is acute.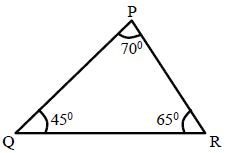

Right-Angled Triangle: A triangle in which one of the angles measures 90° is called a right angled triangle or simply a right triangle. In a right-angled triangle the side opposite to the right angle is called its hypotenuse and the remaining two sides are called its legs.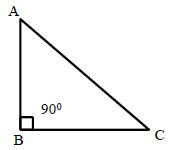

$∆$ABC is a right triangle and ∠B = 90°, AC is the hypotenuse and AB and BC are its legs.
Obtuse-Angled Triangle: A triangle in which one of the angles measures more than 90° but less than 180° is called an obtuse- angled triangle.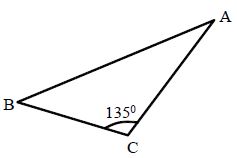

here, $∆$ABC is an obtuse-angled triangle in which ∠C =135°.
Median: A line segment joining a vertex to the mid-point of the opposite side of a triangle is called a median of the triangle. In the below figure, D is the mid-point of side BC of ABC. AD is a median of ABC. A triangle has 3 medians.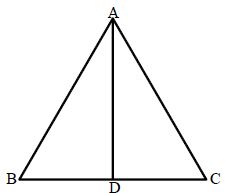

All the three medians of a triangle are concurrent i.e. they intersect at a point
Centroid: The point of intersection (concurrence) of the three medians of a triangle is called its centroid.
In the below figure, the three medians AD, BE and CF intersect at the point G.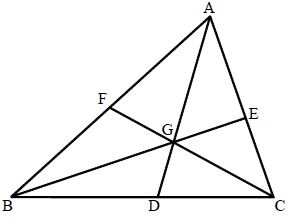

G is the centroid of $∆$ABC
Altitude: The length of perpendicular from a vertex to the opposite side of a triangle is called its altitude and the side on which the perpendicular is being drawn, is called its base. In the below figure, AL$\perp$BC.
So BC is the base and AL is the corresponding altitude of the triangle. A triangle has three altitudes. All the three altitudes of a triangle are concurrent i.e., they intersect at a point.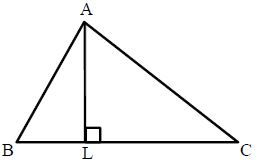

Orthocenter: The point of intersection (concurrence) of the three altitudes of a triangle is called its orthocenter. In the figure below, the three altitudes AL, BM and CN of $∆$ABC intersect at a point H.
Therefore, H is the orthocenter of ABC.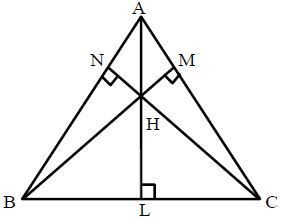

Incentre and Incircle: The point of intersection of the internal bisectors of the angles of a triangle is called its incentre. In the below figure, the bisectors of the internal angles of a $∆$ABC meet at a point I. So, ‘I’ is the incentre of ABC. The incentre of a triangle is the center of a circle which touches all the sides of the triangle and this circle is called the incircle of the triangle. If ID$\perp$BC, then ID is called the radius of the incircle.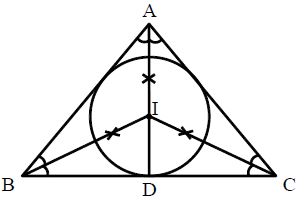

Circumcentre and Circumcircle: The point of intersection of the perpendicular bisectors of the sides of a triangle is called its circumcentre. In the below figure, the perpendicular bisectors of the sides AB, BC and CA of $∆$ABC intersect at a point O. So O is the Circumcentre of the triangle. The circumcentre of a triangle is the centre of a circle which passes through the vertices of the triangle and this circle is called the circumcircle of the triangle. Clearly, OA = OB = OC = radius of circumcircle.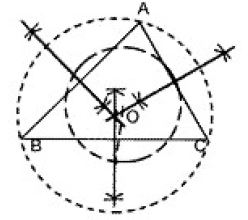

Exterior and Interior Opposite Angles of a Triangle: Let ABC be a triangle one of whose sides BC is produced to D; then ∠ACD is called an exterior angle and the angles ∠A and ∠B are called its interior opposite angles.
Properties of Triangles
• Angle Sum Property: the sum of the angles of a triangle is 180°.
• If two sides of a triangle are equal in length, then the angles opposite to them are of equal measures.
• The sum of any two sides of a triangle is always greater than the third side.
• If 2 sides of a triangle are of an unequal length, then the greater side has the greater angle opposite to it.
• If 1 side of a triangle is produced the exterior angle formed is equal to sum of interior opposite angles.
• In a triangle the exterior angle is always, greater than its interior opposite angle. The sum of exterior angles of a triangle is 360°.

3. QUADRILATERALS
A closed figure bounded by four line segments is called a quadrilateral.
A quadrilateral ABCD has:
• Four vertices, namely A, B, C and D
• Four sides, namely AB, BC, CD and DA
• Four angles, namely ∠A, ∠B, ∠C and ∠D
• Two diagonals, namely AC and BD

Adjacent Sides
Two sides of a quadrilateral having a common end point are called its adjacent or consecutive sides. In the given quadrilateral, (AB, BC), (BC, CD), (CD, DA) and (BA, AD) are four pairs of its adjacent sides.
Opposite Sides
Two sides of a quadrilateral having no common end point are called its opposite sides.
In the given quadrilateral (AB, CD) and (BC, AD) are two pairs of its opposite sides.
Adjacent Angles
Two angles of a quadrilateral having a common arm are called its adjacent or consecutive angles. In the given quadrilateral (∠A, ∠B), (∠B, ∠C), (∠C, ∠D) and (∠D, ∠A) are four pairs of adjacent angles.
Opposite Angles
Two angles of a quadrilateral not having a common arm are called its opposite angles.
In the given quadrilateral (∠A, ∠C) and (∠B, ∠D) are two pairs of its opposite angles.
• The sum of all the angles of a quadrilateral is 360°.
• The sum of exterior angles of a quadrilateral is 360°.
Parallelogram
A quadrilateral in which both pairs of opposite sides are parallel is called a parallelogram.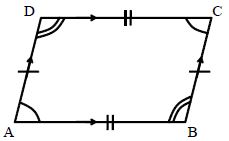

In a Parallelogram:
• Opposite sides are equal
• Opposite angles are equal
• Each diagonal bisects the parallelogram
• If pair of opposite sides of a Quadrilateral are equal and parallel, it is a parallelogram
Rectangle: A parallelogram each of whose angle measures 90° is called a Rectangle.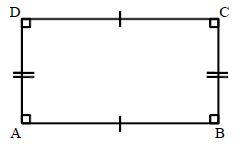

In a Rectangle:
• Opposite sides are equal
• Each angle measure is 90°
• Diagonals are equal
• Diagonals bisect each other
Square: A Rectangle having all sides equal is called a square. If two adjacent sides of a Rectangle are equal, then it is called a Square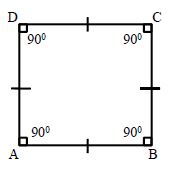

In a Square:
• All sides are equal
• Each angle measures 90°
• Diagonals are equal
• Diagonals bisect each other
• Diagonals intersect at right angles
Rhombus: A parallelogram having all sides equal is called a Rhombus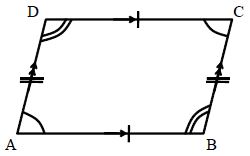

In a Rhombus:
• Opposite sides are parallel
• All sides are equal
• Diagonals bisect each other at right angles
Trapezium: A Quadrilateral in which two opposite sides are parallel is called a trapezium. In diagonal divides proportionally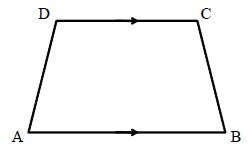

If the non parallel sides of a trapezium are equal. It is known as isosceles-trapezium
Kite: A Quadrilateral in which two pairs of adjacent sides are equal is known as a kite. The longer diagonal bisects the shorter diagonal.

Relation between Quadrilaterals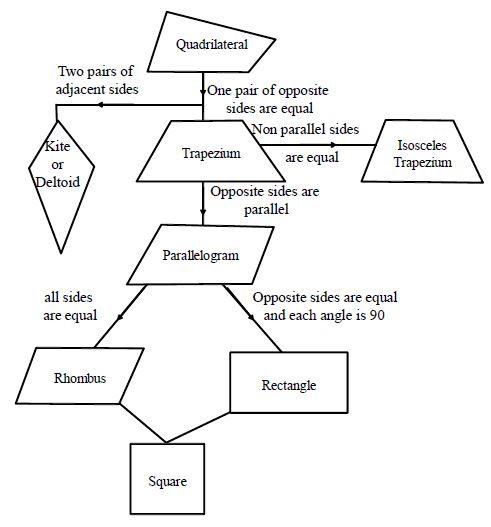

4. POLYGONS
A closed plane figure bounded by three or more line segments is called a polygon. The line segments forming a polygon are called its sides. The point of intersection of two consecutive sides of a polygon is called a vertex.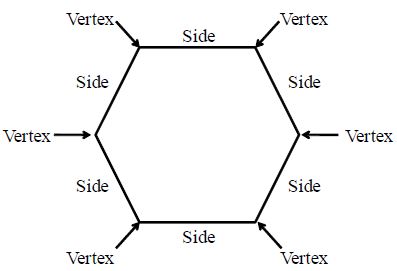

The number of vertices of a polygon is equal to the number of its sides.

A polygon of n-sides is called n-gon. Thus, a polygon of 15 sides is a 15-gon
Diagonal of a Polygon
A line segment joining any two non-consecutive vertices of a polygon is called its diagonal. Thus, in the below figure ABCDE is a polygon and each of the line segments AC, AD, BD, BE and CE is a diagonal of the polygon.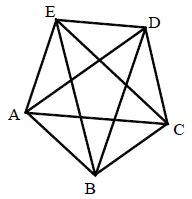

Interior and Exterior Angle of a Polygon:
An angle formed by two consecutive sides of a polygon is called an interior angle or simply an angle of the polygon.

From thi s table, we can see that the number of triangles formed in a polygon is two less than the number of sides. So for a polygon with n sides, the number of triangles formed is n – 2. The angle sum of a polygon with n sides is (n – 2) × 180°. Therefore, S = (n – 2) 180°.
In a regular polygon with n sides each interior angle is equal to $\frac{(n–2)\times 180}{n}$
When each side of a polygon is produced (extended) in a clockwise or anti clockwise direction exterior angles are formed as shown. The sum of the exterior angles of any polygon is 360°.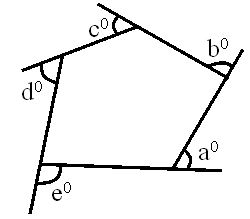

5. THREE DIMENSIONAL FIGURES
Solid State Figures: Objects with length, breadth and height/thickness are called solids.
Cuboid: The solid having the four lateral sides with base and top are in rectangular shape is called ‘cuboid’. The solid formed by six rectangular surfaces is called cuboid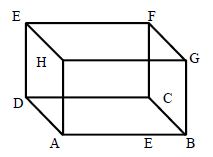

Eg: Bricks, Eraser.
Number of surfaces = 6
Number of vertices = 8
Number of edges = 12
Cube: A Solid formed by the enclosure of six square plane surfaces is called a ‘cube’. Cube has – six faces, eight vertices, twelve edges.

Cylinder: The base and top are circular in shape. The remaining surface is called curved surface.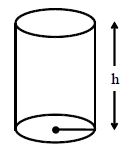

Cone: The base is a circle. Its radius is denoted by r. $\overline{\mathrm{AC}}$ is called slant height and it is denoted by ‘s’. $\overline{\mathrm{AO}}$ is called vertical height and it is denoted by ‘h’. A is called its vertex.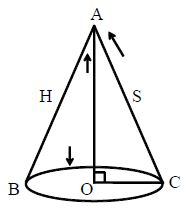

Sphere: Ball, Marbles and balls in the cycle bearings are some of examples of Sphere. In the figure below, ‘O’ is called its ‘centre’.  $\overline{\mathrm{AO}}$ is called its radius. Its surface is called curved surface.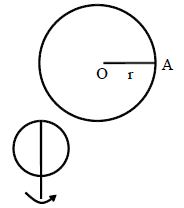

Prism: Triangular Prism. The adjoining figure shows a ‘triangular prism’. It is so called according to the shape of its base (its base is a triangle). It has five surfaces and six vertices.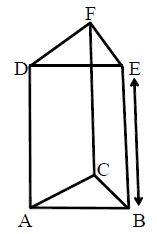

Pyramid: You might have seen the photographs of world famous Pyramids of Egypt. Their base is in square shape and their lateral faces are in triangular shape, such that all the four surfaces meet at one point.
The below figure shows a ‘Pyramid’ with square ABCD as its base.
$\overline{\mathrm{AB}}=\overline{\mathrm{BC}}=\overline{\mathrm{CD}}=\overline{\mathrm{DA}}=\mathrm{a}$, shows the side of its base.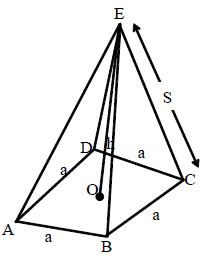

$\overline{\mathrm{EO}}$ = h is called its vertical height. $\overline{\mathrm{EC}}$ = s is called its slant height or lateral height. The four triangular shaped surfaces and square base all together there are five surfaces.
Triangular Pyramid: If a pyramid has triangular base, then it is called a ‘triangular pyramid’. It has three triangular lateral surfaces and one triangular base. All together the triangular pyramid has four triangular faces.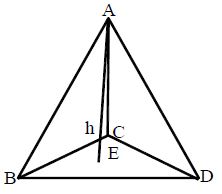

The adjoining figure shows a ‘triangular pyramid’. It’s base is a triangle BCD. Lateral height of each triangle (lateral surfaces) is called the slant height which is denoted as s and the point of meeting all the lateral sides. ‘A’ is called ‘apex or vertex’. $\overline{\mathrm{AE}}$ = h is called height.