Rational Numbers

EXERCISE-1.1

P1. Using appropriate properties find:

(i) $–\frac{2}{3}\times \frac{3}{5}+\frac{5}{2}–\frac{3}{5}\times \frac{1}{6}$

(ii) $\frac{2}{5}\times \left(–\frac{3}{7}\right)–\frac{1}{6}\times \frac{3}{2}+\frac{1}{14}\times \frac{2}{5}\times \frac{1}{6}+\frac{5}{2}$

Sol. (i) $–\frac{2}{3}\times \frac{3}{5}+\frac{5}{2}–\frac{3}{5}\times \frac{1}{6}$

$=–\frac{2}{3}\times \frac{3}{5}–\frac{3}{5}$ (Commutativity)

$=\left(–\frac{3}{5}\right)\times \left(\frac{2}{3}+\frac{1}{6}\right)+\frac{5}{2}$ (Distributivity)

$=\left(–\frac{3}{5}\right)\times \left(\frac{2\times 2+1}{6}\right)+\frac{5}{2}$

$=\left(–\frac{3}{5}\right)\times \left(\frac{5}{6}\right)+\frac{5}{2}$

$=\left(–\frac{3}{6}\right)+\frac{5}{2}=\left(\frac{–3+5\times 3}{6}\right)$

$=\left(\frac{–3+15}{6}\right)=\frac{12}{6}=2$

(ii) $\frac{2}{5}\times \left(–\frac{3}{7}\right)–\frac{1}{6}\times \frac{3}{2}+\frac{1}{14}\times \frac{2}{5}$

$=\frac{2}{5}\times \left(–\frac{3}{7}\right)+\frac{1}{14}\times \frac{2}{5}–\frac{1}{6}\times \frac{3}{2}$ (By Commutativity)

$=\frac{2}{5}\times \left(–\frac{3}{7}+\frac{1}{14}\right)–\frac{1}{4}$ (By Distributivity)

$=\frac{2}{5}\times \left(\frac{–3\times 2+1}{14}\right)–\frac{1}{4}=\frac{2}{5}\times \left(\frac{–5}{14}\right)–\frac{1}{4}$

$=–\frac{1}{7}–\frac{1}{4}=\frac{–4–7}{28}=\frac{–11}{28}$

P2. Write the additive inverse of each of the following:

(i) $\frac{2}{8}$

(ii) $\frac{–5}{9}$

(iii) $\frac{–6}{–5}$

(iv) $\frac{2}{–9}$

(v) $\frac{19}{–6}$

Sol. (i) $\frac{2}{8}$$\Rightarrow$ Additive inverse $=–\frac{2}{8}$

(ii) $\frac{–5}{9}$$\Rightarrow$Additive inverse $=\frac{5}{9}$

(iii) $\frac{–6}{–5}$$\Rightarrow$Additive inverse $=\frac{–6}{5}$

(iv) $\frac{2}{–9}=\frac{–2}{9}$$\Rightarrow$ Additive inverse = $\frac{2}{9}$

(v) $\frac{19}{–6}=\frac{–19}{6}$$\Rightarrow$Additive inverse $=\frac{19}{6}$

P3. Verify that −(−x) = x for.

(i) $\mathrm{x}=\frac{11}{15}$

(ii) $\mathrm{x}=–\frac{13}{17}$

Sol. (i) $\mathrm{x}=\frac{11}{15}$

The additive inverse of $\mathrm{x}=\frac{11}{15}$ is

$–x=–\frac{11}{15}$$\text{ as }\frac{11}{15}+\left(–\frac{11}{15}\right)=0$

This equality $\frac{11}{15}+\left(–\frac{11}{15}\right)=0$ represents that the additive inverse of $–\frac{11}{15}$ is $\frac{11}{15}$ or it can be said that $–\left(–\frac{11}{15}\right)=\frac{11}{15}$ i.e., −(−x) = x

(ii) $x=–\frac{13}{17}$

The additive inverse of is $–x=–\frac{13}{17}$$\text{ as }–\frac{13}{17}+\frac{13}{17}=0$

This equality $–\frac{13}{17}+\frac{13}{17}=0$ represents that the additive inverse of $\frac{13}{17}$ is −$\frac{13}{17}$ i.e., −(−x) = x

P4. Find the multiplicative inverse of the following.

(i) $–$13

(ii) $–\frac{1}{13}$

(iii) $\frac{1}{5}$

(iv) $\frac{–5}{8}\times \frac{–3}{7}$

(v) $–1\times \frac{–2}{5}$

(vi) $–$1

Sol. (i) −13$\Rightarrow$ Multiplicative inverse =$–\frac{1}{13}$

(ii) $\frac{–13}{19}$$\Rightarrow$Multiplicative inverse = $–\frac{19}{13}$

(iii) $\frac{1}{5}$$\Rightarrow$Multiplicative inverse = 5

(iv) $–\frac{5}{8}\times –\frac{3}{7}=\frac{15}{56}$$\Rightarrow$ Multiplicative inverse =$\frac{56}{15}$

(v) $–1\times –\frac{2}{5}=\frac{2}{5}$$\Rightarrow$Multiplicative inverse $=\frac{5}{2}$

(vi) $–$1$\Rightarrow$Multiplicative inverse = $–$1

P5. Name the property under multiplication used in each of the following:

(i) $\frac{–4}{5}\times 1=1\times \frac{–4}{5}=–\frac{4}{5}$

(ii) $–\frac{13}{17}\times \frac{–2}{7}=\frac{–2}{7}\times \frac{–13}{17}$

(iii) $\frac{–19}{29}\times \frac{29}{–19}=1$

Sol. (i) $\frac{–4}{5}\times 1=1\times \frac{–4}{5}=–\frac{4}{5}$

1 is the multiplicative identity.

(ii) Commutativity

(iii) Multiplicative inverse

P6. Multiply $\frac{6}{13}$ by the reciprocal of $\frac{–7}{16}$.

Sol. $\frac{6}{13}\times \left(\text{ Reciprocal of }–\frac{7}{16}\right)$

$=\frac{6}{13}\times –\frac{16}{7}=–\frac{96}{91}$

P7. Tell what property allows you to compute $\left(\frac{1}{3}\times 6\right)\times \frac{4}{3}$.

Sol. Associativity

P8. Is 8/9 the multiplicative inverse of $–\left(1\frac{1}{8}\right)?$ Why or why not?

Sol. If it is the multiplicative inverse, then the product should be 1.

However, here, the product is not 1 as

$\frac{8}{9}\times \left[–\left(1\frac{1}{8}\right)\right]=\frac{8}{9}\times \left(–\frac{9}{8}\right)=–1\ne 1$

P9. Is 0.3 the multiplicative inverse of $3\frac{1}{3}?$? Why or why not?

Sol. $3\frac{1}{3}=\frac{10}{3}$

$0.3\times 3\frac{1}{3}=0.3\times \frac{10}{3}$$=\frac{3}{10}\times \frac{10}{3}=1$

Here, the product is 1. Hence, 0.3 is the multiplicative inverse of $3\frac{1}{3}$ .

P10. Write:

(i) The rational number that does not have a reciprocal.

(ii) The rational numbers that are equal to their reciprocals.

(iii) The rational number that is equal to its negative.

Sol. (i) 0 is a rational number but its reciprocal is not defined.

(ii) 1 and −1 are the rational numbers that are equal to their reciprocals.

(iii) 0 is the rational number that is equal to its negative.

P11. Fill in the blanks.

(i) Zero has __________ reciprocal.

(ii) The numbers __________ and __________ are their own reciprocals

(iii) The reciprocal of − 5 is __________.

(iv) Reciprocal of $\frac{1}{x}$, where $x\ne 0$ is __________.

(v) The product of two rational numbers is always a __________.

(vi) The reciprocal of a positive rational number is __________.

Sol. (i) No

(ii) 1, −1

(iii) $–\frac{1}{5}$

(iv) x

(v) Rational number

(vi) Positive rational number

EXERCISE-1.2

P1. Represent these numbers on the number line.

(i) $\frac{7}{4}$

(ii) $\frac{–5}{6}$

Sol. (i) $\frac{7}{4}$ can be represented on the number line as follows.

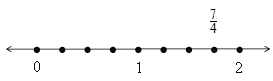
(ii) $\frac{–5}{6}$can be represented on the number line as follows.

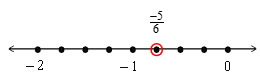
P2. Represent $\frac{–2}{11},\frac{–5}{11},\frac{–9}{11}$ on the number line.

Sol. $\frac{–2}{11},\frac{–5}{11},\frac{–9}{11}$ can be represented on the number line as follows.

P3. Write five rational numbers which are smaller than 2.

Sol. 2 can be represented as $\frac{14}{7}$ .

Therefore, five rational numbers smaller than 2 are $\frac{13}{7},\frac{12}{7},\frac{11}{7},\frac{10}{7},\frac{9}{7}$

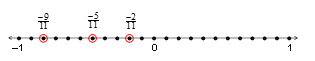
P4. Find ten rational numbers between $\frac{–2}{5}$ and $\frac{1}{2}$.

Sol. $\frac{–2}{5}$and $\frac{1}{2}$ can be represented as $–\frac{8}{20}\text{ and }\frac{10}{20}$ respectively.

Therefore, ten rational numbers between $\frac{–2}{5}$and $\frac{1}{2}$ are

$–\frac{7}{20},–\frac{6}{20},–\frac{5}{20},–\frac{4}{20},–\frac{3}{20},–\frac{2}{20},–\frac{1}{20},0,\frac{1}{20},\frac{2}{20},$

P5. Find five rational numbers between

(i) $\frac{2}{3}\text{ and }\frac{4}{5}$

(ii) $\frac{–3}{2}\text{ and }\frac{5}{3}$

(iii) $\frac{1}{4}\text{ and }\frac{1}{2}$

Sol. (i) $\frac{2}{3}\text{ and }\frac{4}{5}$ can be represented as $\frac{30}{45}\text{ and }\frac{36}{45}$ respectively.

Therefore, five rational numbers between $\frac{2}{3}\text{ and }\frac{4}{5}$ are

$\frac{31}{45},\frac{32}{45},\frac{33}{45},\frac{34}{45},\frac{31}{45}$

(ii) $\frac{–3}{2}\text{ and }\frac{5}{3}$ can be represented as $–\frac{9}{6}\text{ and }\frac{10}{6}$ respectively.

Therefore, five rational numbers between $\frac{–3}{5}\text{ and }\frac{5}{3}$ are $–\frac{8}{6},–\frac{7}{6},–1,–\frac{5}{6},–\frac{4}{6}$

(iii) $\frac{1}{4}\text{ and }\frac{1}{2}$ can be represented as $\frac{8}{32}\text{ and }\frac{16}{32}$ respectively.

Therefore, five rational numbers between $\frac{1}{4}\text{ and }\frac{1}{2}$ are $\frac{9}{32},\frac{10}{32},\frac{11}{32},\frac{12}{32},\frac{13}{32}$

P6. Write five rational numbers greater than − 2.

Sol. −2 can be represented as $–$$\frac{14}{7}$ .

Therefore, five rational numbers greater than −2 are $–\frac{13}{7},–\frac{12}{7},–\frac{11}{7},–\frac{10}{7},–\frac{9}{7}$

P7. Find ten rational numbers between $\frac{3}{5}$and $\frac{3}{4}$.

Sol. $\frac{3}{5}$and $\frac{3}{4}$ can be represented as $\frac{48}{80}\text{ and }\frac{60}{80}$ respectively.

Therefore, ten rational numbers between $\frac{3}{5}$and $\frac{3}{4}$are

$\frac{49}{80},\frac{50}{80},\frac{51}{80},\frac{52}{80},\frac{53}{80},\frac{54}{80},\frac{55}{80},\frac{56}{80},\frac{57}{80},\frac{58}{80}$