Introduction to Trigonometry

EXERCISE-8.1

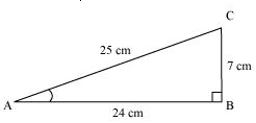
P 1: In ΔABC right angled at B, AB = 24 cm, BC = 7 m. Determine

(i) sin A, cos A (ii) sin C, cos C

Sol. Applying Pythagoras theorem for ΔABC, we obtain

AC² = AB² + BC²

= (24 cm)² + (7 cm)²

= (576 + 49) cm²

= 625 cm²

$\therefore$ AC = $\sqrt{625}$cm = 25 cm

(i) sin A $=\frac{\text{ Side opposite to }\angle \mathrm{A}}{\text{ Htypotenuse }}=\frac{\mathrm{BC}}{\mathrm{AC}}=\frac{7}{5}$

cos A = $\frac{\text{ Side adjacent to }\angle \mathrm{A}}{\text{ Hypotenuse }}=\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{24}{25}$

(ii)

sin C = $\frac{\text{ Side opposite to }\angle \mathrm{C}}{\text{ Hypotenuse }}=\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{24}{25}$

cos C = $\frac{\text{ Side adjacent to }\angle \mathrm{C}}{\text{ Hypotenuse }}=\frac{\mathrm{BC}}{\mathrm{AC}}=\frac{7}{25}$

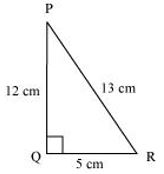
P2. In the given figure find tan P − cot R

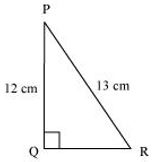
Sol. Applying Pythagoras theorem for ΔPQR, we obtain

PR² = PQ² + QR²

(13 cm)² = (12 cm)² + QR²

169 cm² = 144 cm² + QR²

$\Rightarrow$25 cm² = QR²

$\Rightarrow$ QR = 5 cm

$\mathrm{tanP}=\frac{\text{ Side opposite to }\angle \mathrm{P}}{\text{ side adjacent to }\angle \mathrm{P}}=\frac{\mathrm{QR}}{\mathrm{PQ}}$ = $\frac{5}{12}$

$\mathrm{cotR}=\frac{\text{ side adjacent to }\angle \mathrm{R}}{\text{ Side opposite to }\angle \mathrm{R}}=\frac{\mathrm{QR}}{\mathrm{PQ}}$ = $\frac{5}{12}$

tan P − cot R = $\frac{5}{12}–\frac{5}{12}=0$

P3. If sin A = $\frac{3}{4}$, calculate cos A and tan A.

Sol. Let ΔABC be a right-angled triangle, right-angled at point B.

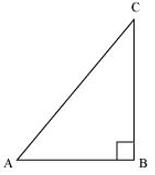
Given that,

$\mathrm{sinA}=\frac{3}{4}$

$\frac{\mathrm{BC}}{\mathrm{AC}}=\frac{3}{4}$

Let BC be 3k.

Therefore, AC will be 4k, where k is a positive integer.

Applying Pythagoras theorem in ΔABC, we obtain

AC² = AB² + BC²

(4k)² = AB² + (3k)²

16k ² − 9k ² = AB²

7k ² = AB²

AB = $\sqrt{7}k$

$\cos A=\frac{\text{ Side adjacent to }\angle A}{\text{ Hypotenuse }}$ $=\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{\sqrt{7}\mathrm{k}}{4\mathrm{k}}=\frac{\sqrt{7}}{4}$

$\tan A=\frac{\text{ Side opposite to }\angle A}{\text{ Side adjacent to }\angle A}$ $=\frac{\mathrm{BC}}{\mathrm{AB}}=\frac{3\mathrm{k}}{\sqrt{7}\mathrm{k}}=\frac{3}{\sqrt{7}}$

P4. Given 15 cot A = 8. Find sin A and sec A

Sol. Consider a right-angled triangle, right-angled at B.

$\cos A=\frac{\text{ Side adjacent to }\angle A}{\text{ Side opposite to }\angle A}=\frac{AB}{BC}$

It is given that, cot A = $\frac{8}{15}$

$\frac{\mathrm{AB}}{\mathrm{BC}}=\frac{8}{15}$

Let AB be 8k. Therefore, BC will be 15k, where k is a positive integer.

Applying Pythagoras theorem in ΔABC, we obtain

AC² = AB² + BC²

= (8k)² + (15k)²

= 64k² + 225k²

= 289k²

AC = 17k

$\sin A=\frac{\text{ Side opposite to }\angle A}{\text{ Hypotenuse }}=\frac{BC}{AC}$ $=\frac{15\mathrm{k}}{17\mathrm{k}}=\frac{15}{17}$

$\sec A=\frac{\text{ Hypotenuse }}{\text{ Side adjacent to }\angle A}$ = $\frac{\mathrm{AC}}{\mathrm{AB}}=\frac{17}{8}$

P5. Given sec θ = $\frac{13}{12}$, calculate all other trigonometric ratios.

Sol. Consider a right-angle triangle ΔABC, right-angled at point B.

$\sec \theta =\frac{\text{ Hypotenuse }}{\text{ Side adjacent to }\angle \theta }$

$\frac{13}{12}=\frac{AC}{AB}$

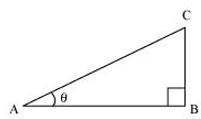
If AC is 13k, AB will be 12k, where k is a positive integer.

Applying Pythagoras theorem in ΔABC, we obtain

(AC)² = (AB)² + (BC)²

(13k)² = (12k)² + (BC)²

169k² = 144k² + BC²

25k² = BC²

BC = 5k

$\sin \theta =\frac{\text{ Side opposite to }\angle \theta }{\text{ Hypotenuse }}=\frac{\mathrm{BC}}{\mathrm{AC}}$ $=\frac{5\mathrm{k}}{13\mathrm{k}}=\frac{5}{13}$

$\cos \theta =\frac{\text{ Side adjacent to }\angle \theta }{\text{ Hypotenuse }}=\frac{\mathrm{AB}}{\mathrm{AC}}$ $=\frac{12\mathrm{k}}{13\mathrm{k}}=\frac{12}{13}$

$\tan \theta =\frac{\text{ Side opposite to }\angle \theta }{\text{ Side adjacent to }\angle \theta }=\frac{\mathrm{BC}}{\mathrm{AB}}$ $=\frac{5\mathrm{k}}{12\mathrm{k}}=\frac{5}{12}$

$\cot \theta =\frac{\text{ Side adjacent to }\angle \theta }{\text{ Side opposite to }\angle \theta }=\frac{\mathrm{AB}}{\mathrm{BC}}$ $=\frac{12\mathrm{k}}{5\mathrm{k}}=\frac{12}{5}$

$cosec\theta =\frac{\text{ Hypotenuse }}{\text{ Side opposite to }\angle \theta }=\frac{\mathrm{AC}}{\mathrm{BC}}$ $=\frac{13\mathrm{k}}{5\mathrm{k}}=\frac{13}{5}$

P6. If ∠A and ∠B are acute angles such that cos A = cos B, then show that ∠A = ∠B.

Sol. Let us consider a triangle ABC in which CD ⊥ AB.

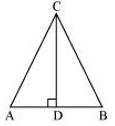
It is given that

cos A = cos B

$\Rightarrow \frac{\mathrm{AD}}{\mathrm{AC}}=\frac{\mathrm{BD}}{\mathrm{BC}}$ … (1)

We have to prove ∠A = ∠B. To prove this, let us extend AC to P such that BC = CP.

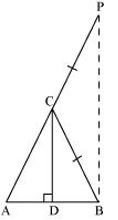
From equation (1), we obtain

$\frac{\mathrm{AD}}{\mathrm{BD}}=\frac{\mathrm{AC}}{\mathrm{BC}}$

$\Rightarrow \frac{\mathrm{AD}}{\mathrm{BD}}=\frac{\mathrm{AC}}{\mathrm{CP}}$

(By construction we have BC = CP)

By using the converse of B.P.T, CD||BP

⇒ ∠ACD = ∠CPB (Corresponding angles) … (3)

And, ∠BCD = ∠CBP (Alternate interior angles) … (4)

By construction, we have BC = CP.

$\therefore$ ∠CBP = ∠CPB (Angle opposite to equal sides of a triangle) … (5)

From equations (3), (4), and (5), we obtain

∠ACD = ∠BCD … (6)

In ΔCAD and ΔCBD,

∠ACD = ∠BCD [Using equation (6)]

∠CDA = ∠CDB [Both 90°]

Therefore, the remaining angles should be equal.

$\therefore$ ∠CAD = ∠CBD

⇒ ∠A = ∠B

Alternatively,

Let us consider a triangle ABC in which CD ⊥ AB.

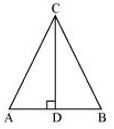
It is given that,

cos A = cos B

$\Rightarrow \frac{\mathrm{AD}}{\mathrm{AC}}=\frac{\mathrm{BC}}{\mathrm{BC}}$

$\Rightarrow \frac{\mathrm{AD}}{\mathrm{BD}}=\frac{\mathrm{AC}}{\mathrm{BC}}$

Let $\Rightarrow \frac{\mathrm{AD}}{\mathrm{BD}}=\frac{\mathrm{AC}}{\mathrm{BC}}=\mathrm{k}$

⇒ AD = k BD … (1)

And, AC = k BC … (2)

Using Pythagoras theorem for triangles CAD and CBD, we obtain

CD² = AC² − AD² … (3)

And, CD² = BC² − BD² … (4)

From equations (3) and (4), we obtain

AC² − AD² = BC² − BD²

⇒ (k BC)² − (k BD)² = BC² − BD²

⇒ k² (BC² − BD²) = BC² − BD²

⇒ k² = 1 ⇒ k = 1

Putting this value in equation (2), we obtain

AC = BC

⇒ ∠A = ∠B (Angles opposite to equal sides of a triangle)

P7. If cot θ = $\frac{\mathbf{7}}{\mathbf{8}}$, evaluate

(i) $\frac{(1+\sin \theta )(1–\sin \theta )}{(1+\cos \theta )(1–\cos \theta )}$

(ii) cot² θ

Sol. Let us consider a right triangle ABC, right-angled at point B.

$\cot \theta =\frac{\text{ Side adjacent to }\angle \theta }{\text{ Side opposite to }\angle \theta }=\frac{7}{8}$

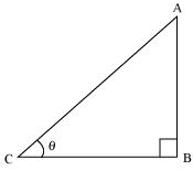
If BC is 7k, then AB will be 8k, where k is a positive integer.

Applying Pythagoras theorem in ΔABC, we obtain

AC² = AB² + BC²

= (8k)² + (7k)²

= 64k² + 49k²

= 113k²

AC = $\sqrt{113}\mathrm{k}$

$\sin \theta =\frac{\text{ Side opposite to }\angle \theta }{\text{ Hypotenuse }}$ $=\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{8\mathrm{k}}{\sqrt{113\mathrm{k}}}=\frac{8}{\sqrt{113}}$

$\cos \theta =\frac{\text{ Side adjacent to }\angle \theta }{\text{ Hypotenuse }}=\frac{\mathrm{BC}}{\mathrm{AC}}$ $=\frac{7k}{\sqrt{113k}}=\frac{7}{\sqrt{113}}$

(i) $\frac{(1+\sin \theta )(1–\sin \theta )}{(1+\cos \theta )(1–\cos \theta )}=\frac{\left(1–{\sin }^2\theta \right)}{\left(1–{\cos }^2\theta \right)}$

$=\frac{1–{\left(\frac{8}{\sqrt{113}}\right)}^2}{1–{\left(\frac{7}{\sqrt{113}}\right)}^2}=\frac{1–\frac{64}{113}}{1–\frac{49}{113}}$

$=\frac{\frac{49}{113}}{\frac{64}{113}}=\frac{49}{64}$

(ii) ${\cot }^2\theta =(\cot \theta {)}^2={\left(\frac{7}{8}\right)}^2=\frac{49}{64}$

P8. If 3 cot A = 4, Check whether  $\frac{1–{\tan }^{2}A}{1+{\tan }^{2}A}={\cos }^{2}A–{\sin }^{2}A$ or not.

Sol. It is given that 3cot A = 4

Or, cot A = $\frac{4}{3}$

Consider a right triangle ABC, right-angled at point B.

$\cot A=\frac{\text{ Side adjacent to }\angle A}{\text{ Side opposite to }\angle A}$ $\Rightarrow \frac{\mathrm{AB}}{\mathrm{BC}}=\frac{4}{3}$

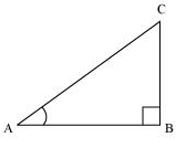
If AB is 4k, then BC will be 3k, where k is a positive integer.

In ΔABC,

(AC)² = (AB)² + (BC)²

= (4k)² + (3k)²

= 16k² + 9k²

= 25k² AC

AC = 5k

$\cos A=\frac{\text{ Side adjacent to }\angle A}{\text{ Hypotenuse }}=\frac{AB}{AC}$ $=\frac{4k}{5k}=\frac{4}{5}$

$\sin A=\frac{\text{ Side opposite to }\angle A}{\text{ Hypotenuse }}=\frac{BC}{AC}$ $=\frac{3k}{5k}=\frac{3}{5}$

$\tan A=\frac{\text{ Side opposite to }\angle A}{\text{ Side adjacent to }\angle A}=\frac{BC}{AB}$ $=\frac{3\mathrm{k}}{4\mathrm{k}}=\frac{3}{4}$

$\frac{1–{\tan }^{2}A}{1+{\tan }^{2}A}=\frac{1–{\left(\frac{3}{4}\right)}^2}{1+{\left(\frac{3}{4}\right)}^2}=\frac{1–\frac{9}{16}}{1+\frac{9}{16}}$ $=\frac{\frac{7}{16}}{\frac{25}{16}}=\frac{7}{25}$

cos² A − sin² A = ${\left(\frac{4}{5}\right)}^2–{\left(\frac{3}{5}\right)}^2$ $=\frac{16}{25}–\frac{9}{25}=\frac{7}{25}$

∴  $\frac{1–{\tan }^{2}A}{1+{\tan }^{2}A}={\cos }^{2}A–{\sin }^{2}A$

P9. In ΔABC, right angled at B. If $\tan A=\frac{1}{\sqrt{3}}$, find the value of

(i) sin A cos C + cos A sin C

(ii) cos A cos C − sin A sin C Sol.

$\tan A=\frac{1}{\sqrt{3}}$

$\frac{\mathrm{BC}}{\mathrm{AB}}=\frac{1}{\sqrt{3}}$

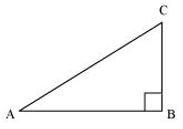
If BC is k, then AB will be $\sqrt{3}k$, where k is a positive integer.

In ΔABC,

AC² = AB² + BC²

= $(\sqrt{3}k{)}^2+(k{)}^2$

= 3k² + k² 

= 4k²

$\therefore$ AC = 2k

$\sin A=\frac{\text{ Side opposite to }\angle A}{\text{ Hypotenuse }}$ $=\frac{\mathrm{BC}}{\mathrm{AC}}=\frac{\mathrm{k}}{2\mathrm{k}}=\frac{1}{2}$

$\cos A=\frac{\text{ Side adjacent to }\angle A}{\text{ Hypotenuse }}$ $=\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{\sqrt{3}\mathrm{k}}{2\mathrm{k}}=\frac{\sqrt{3}}{2}$

$\mathrm{sinC}=\frac{\text{ Side opposite to }\angle \mathrm{C}}{\text{ Hypotenuse }}$ $=\frac{\mathrm{AB}}{\mathrm{AC}}=\frac{\sqrt{3}\mathrm{k}}{2\mathrm{k}}=\frac{\sqrt{3}}{2}$

$\cos C=\frac{\text{ Side adjacent to }\angle C}{\text{ Hypotenuse }}$ $=\frac{\mathrm{BC}}{\mathrm{AC}}=\frac{\mathrm{k}}{2\mathrm{k}}=\frac{1}{2}$

(i) sin A cos C + cos A sin C $=\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)+\left(\frac{\sqrt{3}}{2}\right)\left(\frac{\sqrt{3}}{2}\right)=\frac{1}{4}+\frac{3}{4}$$=\frac{4}{4}=1$

(ii) cos A cos C − sin A sin C $=\left(\frac{\sqrt{3}}{2}\right)\left(\frac{1}{2}\right)–\left(\frac{1}{2}\right)\left(\frac{\sqrt{3}}{2}\right)$ $=\frac{\sqrt{3}}{4}–\frac{\sqrt{3}}{4}=0$

P10. In ΔPQR, right angled at Q, PR + QR = 25 cm and PQ = 5 cm. Determine the values of sin P, cos P and tan P.

Sol. Given that,

PR + QR = 25

PQ = 5

Let PR be x.

Therefore, QR = 25 − x

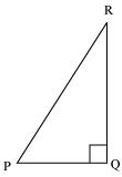
Applying Pythagoras theorem in ΔPQR, we obtain

PR² = PQ² + QR²

x² = (5)² + (25 − x)²

x² = 25 + 625 + x² − 50x

50x = 650

x = 13

Therefore, PR = 13 cm

QR = (25 − 13) cm = 12 cm

$\mathrm{sinP}=\frac{\text{ Side opposite to }\angle \mathrm{P}}{\text{ Hypotenuse }}$ $=\frac{\mathrm{QR}}{\mathrm{PR}}=\frac{12}{13}$

$\cos P=\frac{\text{ Side opposite to }\angle P}{\text{ Hypotenuse }}$ $=\frac{\mathrm{PQ}}{\mathrm{PR}}=\frac{5}{13}$

$\mathrm{tanP}=\frac{\text{ Side opposite to }\angle \mathrm{P}}{\text{ Side adjacent to }\angle \mathrm{P}}$ $=\frac{\mathrm{QR}}{\mathrm{PQ}}=\frac{12}{5}$

P11. State whether the following are true or false. Justify your answer.

(i) The value of tan A is always less than 1.

(ii) sec A = $\frac{12}{5}$ for some value of angle A.

(iii) cos A is the abbreviation used for the cosecant of angle A.

(iv) cot A is the product of cot and A

(v) $\mathit{s}\mathit{i}\mathit{n}\mathit{\theta }\mathbf{=}\frac{\mathbf{4}}{\mathbf{3}}$, for some angle θ

Sol. (i) Consider a ΔABC, right-angled at B.

$\tan A=\frac{\text{ Side opposite to }\angle A}{\text{ Side adjacent to }\angle A}=\frac{12}{5}$

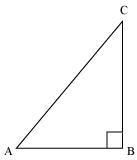
But $\frac{12}{5}>1$

$\therefore$ tan A > 1

So, tan A < 1 is not always true.

Hence, the given statement is false.

(ii) $\sec A=\frac{12}{5}$

$\frac{\text{ Hypotenue }}{\text{ Side adjacent to }\angle \mathrm{A}}=\frac{12}{5}$

$\frac{AC}{AB}=\frac{12}{5}$

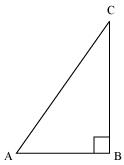
Let AC be 12k, AB will be 5k, where k is a positive integer.

Applying Pythagoras theorem in ΔABC, we obtain

AC² = AB² + BC²

(12k)² = (5k)² + BC²

144k² = 25k² + BC²

BC² = 119k²

BC = 10.9k

It can be observed that for given two sides AC = 12k and AB = 5k,

BC should be such that,

AC − AB < BC < AC + AB

12k − 5k < BC < 12k + 5k

7k < BC < 17 k

However, BC = 10.9k.

Clearly, such a triangle is possible and hence, such value of sec A is possible.

Hence, the given statement is true.

(iii) Abbreviation used for cosecant of angle A is cosec A. And cos A is the abbreviation used for cosine of angle A. Hence, the given statement is false.

(iv) cot A is not the product of cot and A. It is the cotangent of ∠A.

Hence, the given statement is false.

(v) sin θ = $\frac{4}{3}$

We know that in a right-angled triangle,

$\sin \theta =\frac{\text{ Side opposite to }\angle \theta }{\text{ Hypotenuse }}$

In a right-angled triangle, hypotenuse is always greater than the remaining two sides. Therefore, such value of sin θ is not possible.

Hence, the given statement is false

EXERCISE-8.2

P1. Evaluate the following

(i) sin60° cos30° + sin30° cos 60°

(ii) 2tan²45° + cos²30° − sin²60°

(iii) $\frac{\mathbf{c}\mathbf{o}\mathbf{s}\mathbf{45}\mathbf{°}}{\mathbf{s}\mathbf{i}\mathbf{n}\mathbf{30}\mathbf{°}\mathbf{+}\mathbf{cosec}\mathbf{30}\mathbf{°}}$

(iv) $\frac{\mathbf{s}\mathbf{i}\mathbf{n}\mathbf{30}\mathbf{°}\mathbf{+}\mathbf{t}\mathbf{a}\mathbf{n}\mathbf{45}\mathbf{°}\mathbf{–}\mathbf{c}\mathbf{o}\mathbf{s}\mathbf{ec}\mathbf{60}\mathbf{°}}{\mathbf{s}\mathbf{e}\mathbf{c}\mathbf{30}\mathbf{°}\mathbf{+}\mathbf{c}\mathbf{o}\mathbf{s}\mathbf{60}\mathbf{°}\mathbf{+}\mathbf{c}\mathbf{o}\mathbf{t}\mathbf{45}\mathbf{°}}$

(v)  $\frac{\mathbf{5}\mathbf{c}\mathbf{o}{\mathbf{s}}^{\mathbf{2}}\mathbf{60}\mathbf{°}\mathbf{+}\mathbf{4}\mathbf{s}\mathbf{e}{\mathbf{c}}^{\mathbf{2}}\mathbf{30}\mathbf{°}\mathbf{–}\mathbf{t}\mathbf{a}{\mathbf{n}}^{\mathbf{2}}\mathbf{45}\mathbf{°}}{\mathbf{s}\mathbf{i}{\mathbf{n}}^{\mathbf{2}}\mathbf{30}\mathbf{°}\mathbf{+}\mathbf{c}\mathbf{o}{\mathbf{s}}^{\mathbf{2}}\mathbf{30}\mathbf{°}}$

Sol. (i) sin60° cos30° + sin30° cos 60°

$=\left(\frac{\sqrt{3}}{2}\right)\left(\frac{\sqrt{3}}{2}\right)+\left(\frac{1}{2}\right)\left(\frac{1}{2}\right)$ $=\frac{3}{4}+\frac{1}{4}=\frac{4}{4}=1$

(ii) 2tan²45° + cos²30° − sin²60°

$=2(1{)}^2+{\left(\frac{\sqrt{3}}{2}\right)}^2–{\left(\frac{\sqrt{3}}{2}\right)}^2$ $=2+\frac{3}{4}–\frac{3}{4}=2$

(iii) $\frac{\cos 45°}{\sec 30°+cosec30°}$

$=\frac{\frac{1}{\sqrt{2}}}{\frac{2}{\sqrt{3}}+2}=\frac{\frac{1}{\sqrt{2}}}{\frac{2+2\sqrt{3}}{\sqrt{3}}}$

$=\frac{\sqrt{3}}{\sqrt{2}(2+2\sqrt{3})}=\frac{\sqrt{3}}{2\sqrt{2}+2\sqrt{6}}$

$=\frac{\sqrt{3}(2\sqrt{6}–2\sqrt{2})}{(2\sqrt{2}+2\sqrt{6})(2\sqrt{2}–2\sqrt{6})}$

$=\frac{2\sqrt{3}(\sqrt{6}–\sqrt{2})}{(2\sqrt{6}{)}^2–(2\sqrt{2}{)}^2}$

$=\frac{2\sqrt{3}(\sqrt{6}–\sqrt{2})}{24–8}$

$=\frac{2\sqrt{3}(\sqrt{6}–\sqrt{2})}{16}$

$=\frac{\sqrt{18}–\sqrt{16}}{8}=\frac{3\sqrt{2}–\sqrt{6}}{8}$

(iv) $\frac{\sin 30°+\tan 45°–\cos cc60°}{\sec 30°+\cos 60°+\cot 45°}$

$=\frac{\frac{1}{2}+1–\frac{2}{\sqrt{3}}}{\frac{2}{\sqrt{3}}+\frac{1}{2}+1}=\frac{\frac{3}{2}–\frac{2}{\sqrt{3}}}{\frac{3}{2}+\frac{2}{\sqrt{3}}}$

$=\frac{\frac{3\sqrt{3}–4}{2\sqrt{3}}}{\frac{3\sqrt{3}+4}{2\sqrt{3}}}=\frac{(3\sqrt{3}–4)}{(3\sqrt{3}+4)}$

$=\frac{(3\sqrt{3}–4)(3\sqrt{3}–4)}{(3\sqrt{3}+4)(3\sqrt{3}–4)}=\frac{(3\sqrt{3}–4{)}^2}{(3\sqrt{3}{)}^2–(4{)}^2}$

$=\frac{27+16–24\sqrt{3}}{27–16}=\frac{43–24\sqrt{3}}{11}$

(v) $\frac{5{\cos }^{2}60°+4{\sec }^{2}30–{\tan }^{2}45°}{{\sin }^{2}30°+{\cos }^{2}30°}$

$=\frac{5{\left(\frac{1}{2}\right)}^2+4{\left(\frac{2}{\sqrt{3}}\right)}^2–(1{)}^2}{{\left(\frac{1}{2}\right)}^2+{\left(\frac{\sqrt{3}}{2}\right)}^2}$

$=\frac{5\left(\frac{1}{4}\right)+\left(\frac{16}{3}\right)–1}{\frac{1}{4}+\frac{3}{4}}$

$=\frac{\frac{15+64–12}{12}}{\frac{4}{4}}=\frac{67}{12}$

P2. Choose the correct option and justify your choice.

(i) $\frac{\mathbf{2}\mathbf{t}\mathbf{a}\mathbf{n}\mathbf{30}\mathbf{°}}{\mathbf{1}\mathbf{+}\mathbf{t}\mathbf{a}{\mathbf{n}}^{\mathbf{2}}\mathbf{30}\mathbf{°}}\mathbf{=}$

(A). sin60°     (B). cos60°     (C). tan60°     (D). sin30°

(ii) $\frac{\mathbf{1}\mathbf{–}\mathbf{t}\mathbf{a}{\mathbf{n}}^{\mathbf{2}}\mathbf{45}\mathbf{°}}{\mathbf{1}\mathbf{+}\mathbf{t}\mathbf{a}{\mathbf{n}}^{\mathbf{2}}\mathbf{45}\mathbf{°}}\mathbf{=}$

(A). tan90°     (B). 1     (C). sin45°     (D). 0

(iii) sin2A = 2sinA is true when

A = (A). 0°     (B). 30°     (C). 45°     (D). 60°

(iv) $\frac{\mathbf{2}\mathbf{t}\mathbf{a}\mathbf{n}\mathbf{30}\mathbf{°}}{\mathbf{1}\mathbf{–}\mathbf{t}\mathbf{a}{\mathbf{n}}^{\mathbf{2}}\mathbf{30}\mathbf{°}}\mathbf{=}$

(A). cos60°     (B). sin60°     (C). tan60°     (D). sin30°

Sol. (i) $\frac{2\tan 30°}{1+{\tan }^{2}30°}$

$=\frac{2\left(\frac{1}{\sqrt{3}}\right)}{1+{\left(\frac{1}{\sqrt{3}}\right)}^2}=\frac{\frac{2}{\sqrt{3}}}{1+\frac{1}{3}}=\frac{\frac{2}{\sqrt{3}}}{\frac{4}{3}}$

$=\frac{6}{4\sqrt{3}}=\frac{\sqrt{3}}{2}$

Out of the given alternatives, only $\sin 60°=\frac{\sqrt{3}}{2}$

Hence, (A) is correct.

(ii) $\frac{1–{\tan }^{2}45°}{1+{\tan }^{2}45°}$$=\frac{1–(1{)}^2}{1+(1{)}^2}=\frac{1–1}{1+1}=\frac{0}{2}=0$

Hence, (D) is correct.

(iii) Out of the given alternatives, only A = 0° is correct.

As sin 2A = sin 0° = 0

2 sinA = 2sin 0° = 2(0) = 0

Hence, (A) is correct.

(iv) $\frac{2\tan 30°}{1–{\tan }^{2}30°}$

$=\frac{2\left(\frac{1}{\sqrt{3}}\right)}{1–{\left(\frac{1}{\sqrt{3}}\right)}^2}=\frac{\frac{2}{\sqrt{3}}}{1–\frac{1}{3}}=\frac{\frac{2}{\sqrt{3}}}{\frac{2}{3}}=\sqrt{3}$

Out of the given alternatives, only tan 60°

Hence, (C) is correct.

P3. If  $\tan (A+B)=\sqrt{3}$  and $\tan (A–B)=\frac{1}{\sqrt{3}}$; 0° < A + B ≤ 90°, A > B find A and B.

Sol. $\tan (A+B)=\sqrt{3}$

⇒ $\tan (A–B)=\tan 60°$

⇒ A + B = 60 … (1)

$\tan (A–B)=\frac{1}{\sqrt{3}}$

⇒ tan (A − B) = tan30

⇒ A − B = 30 … (2)

On adding both equations, we obtain

2A = 90

⇒ A = 45

From equation (1), we obtain

45 + B = 60

B = 15

Therefore, ∠A = 45° and ∠B = 15°

P4. State whether the following are true or false. Justify your answer.

(i) sin (A + B) = sin A + sin B

(ii) The value of sinθ increases as θ increases

(iii) The value of cos θ increases as θ increases

(iv) sinθ = cos θ for all values of θ

(v) cot A is not defined for A = 0°

Sol. (i) sin (A + B) = sin A + sin B

Let A = 30° and B = 60°

sin (A + B) = sin (30° + 60°) = sin 90° = 1

sin A + sin B = sin 30° + sin 60° $=\frac{1}{2}+\frac{\sqrt{3}}{2}=\frac{1+\sqrt{3}}{2}$

Clearly, sin (A + B) ≠ sin A + sin B

Hence, the given statement is false.

(ii) The value of sin θ increases as θ increases in the interval of 0° < θ < 90° as

sin 0° = 0

$\sin 30°=\frac{1}{2}=0.5$

$\sin 45°=\frac{1}{\sqrt{2}}=0.707$

$\sin 60°=\frac{\sqrt{3}}{2}=0.866$

sin 90° = 1

Hence, the given statement is true.

(iii) cos 0° = 1

$\cos 30°=\frac{\sqrt{3}}{2}=0.866$

$\cos 45°=\frac{1}{\sqrt{2}}=0.707$

$\cos 60°=\frac{1}{2}=0.5$

cos90° = 0

It can be observed that the value of cos θ does not increase in the interval of 0° < θ < 90°.

Hence, the given statement is false.

(iv) sin θ = cos θ for all values of θ.

This is true when θ = 45°

As $\sin 45°=\frac{1}{\sqrt{2}}$

$\cos 45°=\frac{1}{\sqrt{2}}$

It is not true for all other values of θ.

As $\sin 30°=\frac{1}{2}$ and $\cos 30°=\frac{\sqrt{3}}{2}$,

Hence, the given statement is false.

(v) cot A is not defined for A = 0°

As $\cot A=\frac{\cos A}{\sin A}$,

$\cot 0°=\frac{\cos 0°}{\sin 0°}=\frac{1}{0}$ = undefined

Hence, the given statement is true.

EXERCISE-8.3

P1. Evaluate

(I) $\frac{\mathbf{s}\mathbf{i}\mathbf{n}\mathbf{18}\mathbf{°}}{\mathbf{c}\mathbf{o}\mathbf{s}\mathbf{72}\mathbf{°}}$

(II) $\frac{\mathbf{t}\mathbf{a}\mathbf{n}\mathbf{26}\mathbf{°}}{\mathbf{c}\mathbf{o}\mathbf{t}\mathbf{64}\mathbf{°}}$

(III) cos 48° − sin 42°

(IV) cosec 31° − sec 59°

Sol. (I) $\frac{\sin 18°}{\cos 72°}=\frac{\sin \left(90°–72°\right)}{\cos 72°}$

(II) $\frac{\tan 26°}{\cot 64°}=\frac{\tan \left(90°–64°\right)}{\cot 64°}$ $=\frac{\cot 64°}{\cot 64°}=1$

(III) cos 48° − sin 42° = cos (90°− 42°) − sin 42° = sin 42° − sin 42° = 0

(IV) cosec 31° − sec 59°= cosec (90° − 59°) − sec 59°  = sec 59° − sec 59° = 0

P2. Show that

(I) tan 48° tan 23° tan 42° tan 67° = 1

(II) cos 38° cos 52° − sin 38° sin 52° = 0

Sol. (I) tan 48° tan 23° tan 42° tan 67°

= tan (90° − 42°) tan (90° − 67°) tan 42° tan 67°

= cot 42° cot 67° tan 42° tan 67°

= (cot 42° tan 42°) (cot 67° tan 67°)

= (1) (1)

= 1

(II) cos 38° cos 52° − sin 38° sin 52°

= cos (90° − 52°) cos (90°−38°) − sin 38° sin 52°

= sin 52° sin 38° − sin 38° sin 52°

= 0

P3. If tan 2A = cot (A− 18°), where 2A is an acute angle, find the value of A.

Sol. Given that, tan 2A

= cot (A− 18°) cot (90° − 2A)

= cot (A −18°) 90° − 2A

= A− 18° 108° = 3A

A = 36°

P4. If tan A = cot B, prove that A + B = 90°

Sol. Given that,

tan A = cot B

tan A = tan (90° − B)

A = 90° − B

A + B = 90°

P5. If sec 4A = cosec (A− 20°), where 4A is an acute angle, find the value of A.

Sol. Given that,

sec 4A = cosec (A − 20°)

cosec (90° − 4A) = cosec (A − 20°)

90° − 4A= A− 20°

110° = 5A

A = 22°

P6. If A, Band C are interior angles of a triangle ABC then show that$\mathit{s}\mathit{i}\mathit{n}\left(\frac{B+C}{2}\right)\mathbf{=}\mathit{c}\mathit{o}\mathit{s}\frac{\mathbf{A}}{\mathbf{2}}$

Sol. We know that for a triangle ABC,

∠ A + ∠B + ∠C = 180°

∠B + ∠C= 180° − ∠A

$\frac{\angle \mathrm{B}+\angle \mathrm{C}}{2}=90°–\frac{\angle \mathrm{A}}{2}$

$\sin \left(\frac{B+C}{2}\right)\sin \left(90°–\frac{A}{2}\right)$ $=\cos \left(\frac{\mathrm{A}}{2}\right)$

P7. Express sin 67° + cos 75° in terms of trigonometric ratios of angles between 0° and 45°.

Sol. sin 67° + cos 75° = sin (90° − 23°) + cos (90° − 15°) = cos 23° + sin 15°

EXERCISE-8.4

P1. Express the trigonometric ratios sin A, sec A and tan A in terms of cot A.

Sol. We know that,

${cosec}^{2}A=1+{\cot }^{2}A$

$\frac{1}{{cosec}^{2}A}=\frac{1}{1+{\cot }^{2}A}$

${\sin }^{2}A=\frac{1}{1+{\cot }^{2}A}$

$\sin A=\pm \frac{1}{1+{\cot }^{2}A}$

$\sqrt{1+{\cot }^{2}A}$ will always be positive as we are adding two positive quantities.

Therefore, $\sin A=\frac{1}{\sqrt{1+{\cot }^{2}A}}$

We know that, $\mathrm{sinA}=\frac{\mathrm{sinA}}{\mathrm{cosA}}$

However, $\cot A=\frac{\cos A}{\sin A}$

Therefore, $\tan A=\frac{1}{\cot A}$

Also, ${\sec }^{2}A=1+{\tan }^{2}A$ $=1+\frac{1}{{\cot }^{2}A}$ $=\frac{{\cot }^{2}A+1}{{\cot }^{2}A}$

$\sec A=\frac{\sqrt{{\cot }^{2}A+1}}{\cot A}$

P2. Write all the other trigonometric ratios of ∠A in terms of sec A.

Sol. We know that,

$\cos A=\frac{1}{\sec A}$

Also, sin² A + cos² A = 1

sin² A = 1 − cos² A

$\sin A=\sqrt{1–{\left(\frac{1}{\sec A}\right)}^2}$

$\sqrt{\frac{{\sec }^2–1}{{\sec }^{2}A}}=\frac{\sqrt{{\sec }^{2}A–1}}{\sec A}$

tan²A + 1 = sec²A

tan²A = sec²A – 1

$\tan A=\sqrt{{\sec }^{2}A–1}$

$cotA=\frac{\cos A}{\sin A}=\frac{\frac{1}{\sec A}}{\frac{\sqrt{{\sec }^{2}A–1}}{\sec A}}$ $=\frac{1}{\sqrt{{\sec }^{2}A–1}}$

$cosecA=\frac{1}{\sin A}=\frac{\sec A}{\sqrt{{\sec }^{2}A–1}}$

P3. Evaluate

(i)  $\frac{\mathbf{s}\mathbf{i}{\mathbf{n}}^{\mathbf{2}}\mathbf{63}\mathbf{°}\mathbf{+}\mathbf{s}\mathbf{i}{\mathbf{n}}^{\mathbf{2}}\mathbf{27}\mathbf{°}}{\mathbf{c}\mathbf{o}{\mathbf{s}}^{\mathbf{2}}\mathbf{17}\mathbf{°}\mathbf{+}\mathbf{c}\mathbf{o}{\mathbf{s}}^{\mathbf{2}}\mathbf{73}\mathbf{°}}$

(ii) sin25° cos65° + cos25° sin65°

Sol. (i) $\frac{{\sin }^{2}63°+{\sin }^{2}27°}{{\cos }^{2}17°+{\cos }^{2}73°}$

$=\frac{{\left[\sin \left(90°–27°\right)\right]}^2+{\sin }^{2}27°}{{\left[\cos \left(90°–73°\right)\right]}^2+{\cos }^{2}73°}$

$=\frac{{\left[\cos 27°\right]}^2+{\sin }^{2}27°}{{\left[\sin 73°\right]}^2+{\cos }^{2}73°}$

$=\frac{{\cos }^{2}27°+{\sin }^{2}27°}{{\sin }^{2}73°+{\cos }^{2}73°}$

= $\frac{1}{1}$ (As sin²A + cos²A = 1) = 1

(ii) sin25° cos65° + cos25° sin65°

$=\left(\sin 25°\right)\left\{\cos 90°–25°\right\}+$ $\cos 25°\left\{\sin \left(90°–25°\right)\right\}$

$=\left(\sin 25°\right)\left(\sin 25°\right)+\left(\cos 25°\right)\left(\cos 25°\right)$

= sin²25° + cos²25°

= 1 (As sin²A + cos²A = 1)

P4. Choose the correct option. Justify your choice.

(i) 9 sec² A − 9 tan² A =

(A) 1     (B) 9     (C) 8     (D) 0

(ii) (1+tan θ + sec θ) (1 + cot θ − cosec θ)

(A) 0     (B) 1     (C) 2     (D) −1

(iii) (secA + tanA) (1 − sinA) =

(A) secA     (B) sinA     (C) cosecA     (D) cosA

(iv)  $\frac{\mathbf{1}\mathbf{+}\mathbf{t}\mathbf{a}{\mathbf{n}}^{\mathbf{2}}\mathbf{A}}{\mathbf{1}\mathbf{+}\mathbf{c}\mathbf{o}{\mathbf{t}}^{\mathbf{2}}\mathbf{A}}$

(A) sec² A     (B) −1     (C) cot² A     (D) tan² A

Sol. (i) 9 sec²A − 9 tan²A

= 9 (sec²A − tan²A)

= 9 (1) [As sec² A − tan² A = 1]

= 9

Hence, alternative (B) is correct.

(ii) (1 + tan θ + sec θ) (1 + cot θ − cosec θ)

$=\left(1+\frac{\sin \theta }{\cos \theta }+\frac{1}{\cos \theta }\right)\left(1+\frac{\cos \theta }{\sin \theta }–\frac{1}{\sin \theta }\right)$

$=\left(\frac{\cos \theta +\sin \theta +1}{\cos \theta }\right)\left(\frac{\sin \theta +\cos \theta –1}{\sin \theta }\right)$

$=\frac{(\sin \theta +\cos \theta {)}^2–(1{)}^2}{\sin \theta \cos \theta }$

$=\frac{{\sin }^2\theta +{\cos }^2\theta +2\sin \theta \cos \theta –1}{\sin \theta \cos \theta }$

$=\frac{1+2\sin \theta \cos \theta –1}{\sin \theta \cos \theta }=2$

Hence, alternative (C) is correct.

(iii) (secA + tanA) (1 − sinA)

$=\left(\frac{1}{\cos A}+\frac{\sin A}{\cos A}\right)(1–\sin A)$

$=\frac{1–{\sin }^{2}A}{\cos A}=\frac{{\cos }^{2}A}{\cos A}$

= cosA

Hence, alternative (D) is correct.

(iv) $\frac{1+{\tan }^{2}A}{1+{\cot }^{2}A}$

$=\frac{1+\frac{{\sin }^{2}A}{{\cos }^{2}A}}{1+\frac{{\cos }^{2}A}{{\sin }^{2}A}}$

= $\frac{\frac{{\cos }^{2}A+{\sin }^{2}A}{{\cos }^{2}A}}{\frac{{\sin }^{2}A+{\cos }^{2}A}{{\sin }^{2}A}}$

$=\frac{\frac{1}{{\cos }^{2}A}}{\frac{1}{{\sin }^{2}A}}$

$=\frac{{\sin }^{2}A}{{\cos }^{2}A}={\tan }^{2}A$

Hence, alternative (D) is correct.

P5. Prove the following identities, where the angles involved are acute angles for which the expressions are defined.

(i) $(cosec\theta –\cot \theta {)}^2=\frac{1–\cos \theta }{1+\cos \theta }$

(ii) $\frac{\cos A}{1+\sin A}+\frac{1+\sin A}{\cos A}=2\sec A$

(iii) $\frac{\tan \theta }{1–\cot \theta }+\frac{\cot \theta }{1–\tan \theta }=1+\sec \theta cosec\theta$

(iv) $\frac{1+\sec A}{\sec A}=\frac{{\sin }^{2}A}{1–\cos A}$

(v) $\frac{\cos A–\sin A+1}{\cos A+\sin A–1}=cosecA+\cot A$

(vi) $\sqrt{\frac{1+\mathrm{sinA}}{1–\mathrm{sinA}}}=\mathrm{secA}+\mathrm{tanA}$

(vii) $\frac{\sin \theta –2{\sin }^3\theta }{2\cos \theta –\cos \theta }=\tan \theta$

(viii) $(\sin A+cosecA{)}^2+(\cos A+\sec A{)}^2$ $=7+{\tan }^{2}A+{\cot }^{2}A$

(ix) $(cosecA–\sin A)(\sec A–\cos A)$$=\frac{1}{\tan A+\cot A}$

(x) $\left(\frac{1+{\tan }^{2}A}{1+{\cot }^{2}A}\right)$ $={\left(\frac{1–\mathrm{tanA}}{1–\mathrm{cotA}}\right)}^2={\tan }^2 \mathrm{A}$

Sol. (i) $(cosec\theta –\cot \theta {)}^2=\frac{1–\cos \theta }{1+\cos \theta }$

$\mathrm{L}.\mathrm{H}.\mathrm{S}=(cosec\theta –\cot \theta {)}^2$

$={\left(\frac{1}{\sin \theta }–\frac{\cos \theta }{\sin \theta }\right)}^2$

$=\frac{(1–\cos \theta {)}^2}{(\sin \theta {)}^2}=\frac{(1–\cos \theta {)}^2}{{\sin }^2\theta }$

$=\frac{(1–\cos \theta {)}^2}{1–{\cos }^2\theta }=\frac{(1–\cos \theta {)}^2}{(1–\cos \theta )(1+\cos \theta )}$

$=\frac{1–\cos \theta }{1+\cos \theta }$

= R.H.S

(ii) $\frac{\cos A}{1+\sin A}+\frac{1+\sin A}{\cos A}=2\sec A$

L.H.S = $\frac{\cos A}{1+\sin A}+\frac{1+\sin A}{\cos A}$

= $\frac{{\cos }^{2}A+(1+\sin A{)}^2}{(1+\sin A)\left(\cos A\right)}$

= $\frac{{\cos }^{2}A+1+{\sin }^{2}A+2\sin A}{(1+\sin A)\left(\cos A\right)}$

= $\frac{{\sin }^{2}A+{\cos }^{2}A+1+2\sin A}{(1+\sin A)\left(\cos A\right)}$

$=\frac{1+1+2\mathrm{sinA}}{(1+\mathrm{sinA})\left(\mathrm{cosA}\right)}$

= $\frac{2}{\cos A}=2\sec A$

= R.H.S

(iii) $\frac{\tan \theta }{1–\cot \theta }+\frac{\cot \theta }{1–\tan \theta }=1+\sec \theta cosec\theta$

L.H.S = $\frac{\tan \theta }{1–\cot \theta }+\frac{\cot \theta }{1–\tan \theta }$

$=\frac{\frac{\sin \theta }{\cos \theta }}{1–\frac{\cos \theta }{\sin \theta }}+\frac{\frac{\cos \theta }{\sin \theta }}{1–\frac{\sin \theta }{\cos \theta }}$

$=\frac{\frac{\sin \theta }{\cos \theta }}{\frac{\sin \theta –\cos \theta }{\sin \theta }}+\frac{\frac{\cos \theta }{\sin \theta }}{\frac{\cos \theta –\sin \theta }{\cos \theta }}$

$=\frac{{\sin }^2\theta }{\cos \theta (\sin \theta –\cos \theta )}–\frac{{\cos }^2\theta }{\sin \theta (\sin \theta –\cos \theta )}$

$=\frac{1}{(\sin \theta –\cos \theta )}\left[\frac{{\sin }^2\theta }{\cos \theta }–\frac{{\cos }^2\theta }{\sin \theta }\right]$

$=\frac{1}{(\sin \theta –\cos \theta )}\left[\frac{{\sin }^2\theta –{\cos }^2\theta }{\sin \theta \cos \theta }\right]$

$=\frac{1}{(\sin \theta –\cos \theta )}\left[\frac{(\sin \theta –\cos \theta )\left({\sin }^2\theta +{\cos }^2\theta +\sin \theta \cos \theta \right)}{\sin \theta \cos \theta }\right]$

$=\left(\frac{1+\sin \theta \cos \theta }{\left(\sin \theta \cos \theta \right)}\right)$

= 1+ secθ cosecθ

= R.H.S.

(iv) $\frac{1+\sec A}{\sec A}=\frac{{\sin }^{2}A}{1–\cos A}$

L.H.S = $\frac{1+\sec A}{\sec A}=\frac{1+\frac{1}{\cos A}}{\frac{1}{\cos A}}$

$=\frac{\frac{\cos A+1}{\cos A}}{\frac{1}{\cos A}}=(\cos A+1)$

$=\frac{(1–\mathrm{cosA})(1+\mathrm{cosA})}{(1–\mathrm{cosA})}$

$=\frac{1–{\cos }^{2}A}{1–\cos A}=\frac{{\sin }^{2}A}{1–\cos A}$

Using the identity cosec²  = 1 + cot² A,

L.H.S = $\frac{\cos A–\sin A+1}{\cos A+\sin A–1}$

$=\frac{\frac{\cos A}{\sin A}–\frac{\sin A}{\sin A}+\frac{1}{\sin A}}{\frac{\cos A}{\sin A}+\frac{\sin A}{\sin A}+\frac{1}{\sin A}}$

$=\frac{\cot A–1+cosecA}{\cot A+1–\mathrm{cossec}A}$

$=\frac{\left\{\right(\cot A)–(1–cosecA\left)\right\} \left\{\right(\cot A)–(1–cosecA\left)\right\}}{\left\{\right(\cot A)+(1–cosecA\left)\right\} \left\{\right(\cot A)–(1–cosecA\left)\right\}}$

$=\frac{(\cot A–1+\cos ecA{)}^2}{(\cot A{)}^2–(1–cosecA{)}^2}$             ${\cot }^{2}A+1+{cosec}^{2}A–2\cot A$

$=\frac{–2\cos ecA+2\cot AcosecA}{{\cot }^{2}A–\left(1+\cos e{c}^{2}A–2\cos ecA\right)}$

$=\frac{2\cos {ec}^{2}A+2\cot AcosecA–2\cot A–2cosecA}{{\cot }^{2}A–1–\cos e{c}^{2}A+2cosecA}$

$=\frac{2{cosec}^{2}A(cosecA+\cot A)–2(\cot A+cosecA)}{{\cot }^{2}A–{cosec}^{2}A–1+2cosecA}$

$=\frac{(cosecA+\cot A)(2cosecA–2)}{–1–1+2cosecA}$

$=\frac{(cosecA+\cot A)(2cosecA–1)}{(2cosecA–2)}$

= cosec A + cot A

= R.H.S

(vi) $\sqrt{\frac{1+\mathrm{sinA}}{1–\mathrm{sinA}}}=\mathrm{secA}+\mathrm{tanA}$

L.H.S = $\sqrt{\frac{1+\sin A}{1–\sin A}}$

$=\sqrt{\frac{(1+\mathrm{sinA})(1+\mathrm{sinA})}{(1–\mathrm{sinA})(1–\mathrm{sinA})}}$

$=\frac{(1+\mathrm{sinA})}{\sqrt{\left(1–{\sin }^2 \mathrm{A}\right)}}=\frac{1+\mathrm{sinA}}{\sqrt{{\cos }^2 \mathrm{A}}}$

$=\frac{1+\mathrm{sinA}}{\mathrm{cosA}}=\mathrm{secA}+\mathrm{tanA}=\mathrm{R}·\mathrm{H}.\mathrm{S}$

(vii) $\frac{\sin \theta –2{\sin }^3\theta }{2\cos \theta –\cos \theta }=\tan \theta$

L.H.S = $\frac{\sin \theta –2{\sin }^3\theta }{2\cos \theta –\cos \theta }$

$=\frac{\sin \theta \left(1–2{\sin }^2\theta \right)}{\cos \theta \left(2{\cos }^2\theta –1\right)}$

$=\frac{\sin \theta \times \left(1–2{\sin }^2\theta \right)}{\cos \theta \times \left(2\left(1–{\sin }^2\theta \right)–1\right)}$

$=\frac{\sin \theta \times \left(1–2{\sin }^2\theta \right)}{\cos \theta \times \left(1–2{\sin }^2\theta \right)}$

$=\tan \theta =\mathrm{R}.\mathrm{H}.\mathrm{S}$

(viii) $(\sin A+cosecA{)}^2+(\cos A+\sec A{)}^2$

$=7+{\tan }^{2}A+{\cot }^{2}A$

L.H.S = $(\sin A+cosecA{)}^2+(\cos A+\sec A{)}^2$

$={\sin }^{2}A+{cosec}^{2}A+2\sin A\cos ecA+{\cos }^{2}A+{\sec }^{2}A+2\cos A\sec A$

$=\left({\sin }^{2}A+{\cos }^{2}A\right)+\left({cosec}^{2}A+{\sec }^{2}A\right)$ $+2\mathrm{sinA}\left(\frac{1}{\mathrm{sinA}}\right)+2\mathrm{cosA}\left(\frac{1}{\mathrm{cosA}}\right)$

$=\left(1\right)+\left(1+{\cot }^{2}A+1+{\tan }^{2}A\right)+\left(2\right)+\left(2\right)$

$=7+{\tan }^{2}A+{\cot }^{2}A$ = R.H.S

(ix) $(cosecA–\sin A)(\sec A–\cos A)$$=\frac{1}{\tan A+\cot A}$

L.H.S = $(cosecA–\sin A)(\sec A–\cos A)$

$=\left(\frac{1}{\mathrm{sinA}}–\mathrm{sinA}\right)\left(\frac{1}{\mathrm{cosA}}–\mathrm{cosA}\right)$

$=\left(\frac{1–{\sin }^{2}A}{\sin A}\right)\left(\frac{1–{\cos }^{2}A}{\cos A}\right)$

$=\frac{\left({\cos }^{2}A\right)\left({\sin }^{2}A\right)}{\sin A\cos A}$

$=\sin A\cos A$

R.H.S = $\frac{1}{\tan A+\cot A}$

$=\frac{1}{\frac{\mathrm{sinA}}{\mathrm{cosA}}+\frac{\mathrm{cosA}}{\mathrm{sinA}}}$

$=\frac{1}{\frac{{\sin }^2 \mathrm{A}+{\cos }^2 \mathrm{A}}{\mathrm{sinAcosA}}}$

$=\frac{\sin A\cos A}{{\sin }^{2}A+{\cos }^{2}A}$

$=\sin A\cos A$

Hence, L.H.S = R.H.S

(x) $\left(\frac{1+{\tan }^{2}A}{1+{\cot }^{2}A}\right)$$={\left(\frac{1–\tan A}{1–\cot A}\right)}^2={\tan }^{2}A$

= $\frac{1+{\tan }^{2}A}{1+{\cot }^{2}A}$ $=\frac{1+\frac{{\sin }^{2}A}{{\cos }^{2}A}}{1+\frac{{\cos }^{2}A}{{\sin }^{2}A}}$ $=\frac{\frac{{\cos }^{2}A+{\sin }^{2}A}{{\cos }^{2}A}}{\frac{{\sin }^{2}A+{\cos }^{2}A}{{\sin }^{2}A}}$

$=\frac{\frac{1}{{\cos }^{2}A}}{\frac{1}{{\sin }^{2}A}}$ $=\frac{{\sin }^{2}A}{{\cos }^{2}A}={\tan }^{2}A$

${\left(\frac{1–\tan A}{1–\cot A}\right)}^2=\frac{1+{\tan }^{2}A–2\tan A}{1+{\cot }^{2}A–2\cot A}$

$=\frac{{\sec }^{2}A–2\tan A}{{cosec}^{2}A–2\cot A}$

$=\frac{\frac{1}{{\cos }^{2}A}–\frac{2\sin A}{\cos A}}{\frac{1}{{\sin }^{2}A}–\frac{2\cos A}{\sin A}}$$=\frac{\frac{1–2\sin A\cos A}{{\cos }^{2}A}}{\frac{1–2\sin A\cos A}{{\sin }^{2}A}}$

$=\frac{{\sin }^{2}A}{{\cos }^{2}A}={\tan }^{2}A$