Kinetic Theory

1. INTRODUCTION

We have studied the mechanics of single particles. When we approach the mechanics associated with the many particles in system such as gases, liquids and solids, we are faced with analyzing the dynamics of a huge number of particles. The dynamics of such many particles systems is called statical mechanics.

The kinetic theory that we study is special aspect of statical mechanics of large number of particles. We begin with the simplest model for a mono atomic ideal gas, a dilute gas whose particles are single atoms rather than molecules. Macroscopic variables of a gas are pressure, volume and temperature and microscopic properties are speed of gas molecules, momentum of molecules etc. Kinetic theory of gases relates the microscopic properties to macroscopic properties. The kinetic theory of gases attempts to develop a matter of the molecular behavior which should result in the observed behavior of an ideal gas. 

2. ASSUMPTIONS OF KINETIC THEORY OF GASES

1. All gases are made of molecules moving randomly in all directions.

2. The size of a molecule is much smaller than the average separation between the molecules.

3. The molecules exert no force on each other or on the walls of the container except during collision.

4. All collisions between two molecules or between a molecule and a wall are perfectly elastic. Also, the time spent during a collision is negligibly small.

5. The molecules obey Newton’s law of motion.

6. When a gas is left for sufficient time, it comes to a steady state. The density and the distribution of molecules with different velocities are independent of position, direction and time. This assumption may be justified if number of molecules is very large.

3. THE PRESSURE OF AN IDEAL GAS

Consider an ideal gas consisting of N molecules in a container of volume ‘v’. The container is a cube with edges of length ‘d’. Consider the collision of one molecule moving with a velocity ‘v’ towards the right hand face of the cube.

The molecules has velocity components $v_x, v_y$,  and v

Then, $(\overline{\mathrm{v}}{)}^2={\left({\overline{\mathrm{v}}}_{\mathrm{x}}\right)}^2+{\left({\overline{\mathrm{v}}}_{\mathrm{y}}\right)}^2+{\left({\overline{\mathrm{v}}}_{\mathrm{z}}\right)}^2$

Because, the motion is completely random, the average values ${\left({\overline{\mathrm{v}}}_{\mathrm{x}}\right)}^2, {\left({\overline{\mathrm{v}}}_{\mathrm{y}}\right)}^2\text{ and }{\left({\overline{\mathrm{v}}}_{\mathrm{z}}\right)}^2$ are equal to each other.  

$(\overline{\mathrm{v}}{)}^2=3{\left({\overline{\mathrm{v}}}_{\mathrm{x}}\right)}^2=3{\left({\overline{\mathrm{v}}}_{\mathrm{y}}\right)}^2=3{\left({\overline{\mathrm{v}}}_{\mathrm{z}}\right)}^2$

or, ${\left({\overline{v}}_x\right)}^2=\frac{1}{3}(\overline{v}{)}^2$

Therefore, ${\mathrm{F}}_{\text{wall }}=\frac{\mathrm{N}}{3}\left(\frac{\mathrm{m}(\overline{\mathrm{v}}{)}^2}{ \mathrm{d}}\right)$

and pressure on the wall,

$\mathrm{p}=\frac{{\mathrm{F}}_{\text{wall }}}{\mathrm{A}}=\frac{{\mathrm{F}}_{\text{wall }}}{{\mathrm{d}}^2}$

$=\frac{1}{3}\left(\frac{\mathrm{N}}{{\mathrm{d}}^3} \mathrm{m}(\overline{\mathrm{v}}{)}^2\right)=\frac{1}{3}\left(\frac{\mathrm{N}}{\mathrm{v}}\right)\left(\mathrm{m}(\overline{\mathrm{v}}{)}^2\right)$

$=\frac{2}{3}\left(\frac{\mathrm{N}}{\mathrm{v}}\right)\left(\frac{1}{2}\mathrm{m}(\overline{\mathrm{v}}{)}^2\right)$

= $\frac{2}{3}$(Number of molecules per unit volume)$\times$(Average transalational kinetic energy)

4. ABSOLUTE OR KELVIN SCALE OF TEMPERATURE

When a gas is heated from $0°C$ to $t°C$ at constant pressure, let us assume that its volume increases from $v_0$ to $v_t$.

If ‘$\alpha$’ is the volume coefficient of the gas, then, ${\mathrm{v}}_{\mathrm{t}}={\mathrm{v}}_0(1+\mathrm{\alpha t})={\mathrm{v}}_0\left(1+\frac{\mathrm{t}}{273.15}\right)$

$=v_0\left(\frac{273.15+t}{273.15}\right)$

$=v_0\left(\frac{T}{273.15}\right)$

$\therefore {\mathrm{v}}_{\mathrm{t}} \propto \mathrm{T}$at constant pressure

But we know that, $\alpha =\beta =\frac{1}{273.15}$

Also, when t = -273.15$°C$, T = 0 and $v_t=0$. i.e. when the temperature of a gas is decreased below , the volume or pressure of gas gradually decreases and become zero.

At still lower temperature, the volume has to become negative which is impossible. Hence, it is established that the minimum temperature theoretically obtainable is only $T=273.15°C$, which is called absolute zero of temperature. The scale developed with this as minimum temperature is known as absolute scale of temperature. Temperature in this scale is represented by T. The unit of temperature is Kelvin and it is represented by symbol K.

i.e. $\mathrm{TK}=273.15+{\mathrm{t}}^0\mathrm{C}$

for example, ${30}^0\mathrm{C}\to {30}^0\mathrm{C}+273=303\mathrm{k}$

Note: Temperature values in this scale do not depend upon any specific properties of gases.  

Kinetic energy of an ideal gas

From the result obtained in pressure as an ideal gas is

$\mathrm{p}=\frac{2}{3}\left(\frac{\mathrm{N}}{\mathrm{v}}\right)\left(\frac{1}{2} \mathrm{m}(\overline{\mathrm{v}}{)}^2\right)$

  or,       $\mathrm{pv}=\frac{2}{3} \mathrm{N}\left(\frac{1}{2} \mathrm{m}(\overline{\mathrm{v}}{)}^2\right)$   …….. (1)

Let us now, compare it with the ideal gas equation

pv = nRT  …….. (2)

From equation (1) and (2), we get,  

$\mathrm{nRT}=\frac{2}{3} \mathrm{N}\left(\frac{1}{2} \mathrm{m}(\overline{\mathrm{v}}{)}^2\right)$

Here,  $\mathrm{n}=\frac{\mathrm{N}}{\mathrm{NA}}$       ($\therefore$ NA = Avogadro number)

Then, $\left(\frac{1}{2}\mathrm{m}(\overline{\mathrm{v}}{)}^2\right)=\frac{3}{2}{\left(\frac{\mathrm{R}}{\mathrm{NA}}\right)}^{\mathrm{T}}=\frac{3}{2}\mathrm{kT}$

Where, k = Boltzmann’s constant = $1.38\times {10}^{–23} \mathrm{J}/\mathrm{k}$

i.e. the average translation kinetic energy per molecule = $(3/2)\mathrm{kT}$

Now, the total translation K.E. of one mole of an ideal gas =   $(\mathrm{K}.\mathrm{E}.{)}_{\text{trans. }}=(3/2)\mathrm{RT}$

Root mean square speed

The square root of ${\mathrm{v}}^{–2}$ is called the root mean square (rms) speed of the molecules.

Then, ${\mathrm{v}}_{\mathrm{rms}}=\sqrt{{\mathrm{v}}^{–2}}=\sqrt{\frac{3\mathrm{kT}}{\mathrm{m}}}$      $\left(\because \left(\frac{1}{2}\mathrm{m}(\overline{\mathrm{v}}{)}^2\right)=\frac{3}{2}\mathrm{kT}\right)$

Using, $\mathrm{k}=\frac{\mathrm{R}}{\mathrm{NA}}$    and, ${\mathrm{mN}}_{\mathrm{A}}=\mathrm{M}$

Now,  ${\mathrm{V}}_{\mathrm{rms}}=\sqrt{\frac{3\mathrm{RT}}{\mathrm{M}}}=\sqrt{\frac{3\mathrm{p}}{\mathrm{\rho }}}$     $\left[\text{ From gas law, }RT=\frac{pM}{\rho }\right]$

5. MEAN SPEED OR AVERAGE SPEED

The particles of a gas have a range of speeds. The average speed is found by taking the average of speeds of all the particles at a given instant. Remember that the speed is positive, since it is magnitude of the velocity.  

${\mathrm{v}}_{\mathrm{av}}=\frac{{\mathrm{v}}_1+{\mathrm{v}}_2+\dots ..+{\mathrm{v}}_{\mathrm{n}}}{\mathrm{N}}$

Then, ${\mathrm{v}}_{\mathrm{av}}=\sqrt{\frac{8\mathrm{kT}}{\mathrm{\pi m}}}=\sqrt{\frac{8\mathrm{RT}}{\mathrm{\pi M}}}=\sqrt{\frac{8\mathrm{p}}{\mathrm{\pi \rho }}}$

Note:

1. In the above expressions of  ${\mathrm{V}}_{\mathrm{rms}}\text{ and }{\mathrm{V}}_{\mathrm{av}}$ , M is the molar mass in kilograms per mole

2. Most probable speed = ${\mathrm{v}}_{\mathrm{mp}}=\sqrt{\frac{2\mathrm{RT}}{\mathrm{M}}}$

3. ${\mathrm{v}}_{\mathrm{rms}}>{\mathrm{v}}_{\mathrm{av}}>{\mathrm{v}}_{\mathrm{mp}}$

4. ${\mathrm{v}}_{\mathrm{rms}}:{\mathrm{v}}_{\mathrm{av}}:{\mathrm{v}}_{\mathrm{mp}}=\sqrt{3}:\sqrt{2.5}:\sqrt{2}$

6. DEDUCTION FROM KINETIC THEORY

Boyle’s law

At a given temperature, the pressure of a given mass of a gas is inversely proportional to its volume. This is known as Boyle’s law.

We have, $\mathrm{pv}=\frac{1}{3}.\mathrm{N}\left({\mathrm{mv}}_{\mathrm{rms}}^2\right)$

Here, ${\mathrm{v}}_{\text{rms }}^2\propto$. For a given mass of gas, m and N are constants.

Thus,    pv = constant

or,  $\mathrm{p}\propto \frac{1}{\mathrm{v}}$

Charle’s law

i) Constant pressure law

“At constant pressure, the volume of a given mass of a gas is directly proportional to the absolute temperature”. This is known as charle’s law.

We have,   $\mathrm{pv}=\frac{1}{3} \mathrm{N}\left({\mathrm{mv}}_{\mathrm{rms}}^2\right)$

If p is constant

$\mathrm{v}\propto {\mathrm{v}}_{\mathrm{rms}}^2$ and ${\mathrm{v}}_{\mathrm{rms}}^2\propto \mathrm{T}$

We get, $\mathrm{v}\propto \mathrm{T}$

ii) Constant volume law

“At constant volume, the pressure of a given mass of a gas is directly proportional to the absolute scale of temperature”. This is known as charle’s law of pressure.

If ‘v’ is constant then,

$\text{ pv }=\frac{1}{3}{\mathrm{Nmv}}_{\text{rms }}^2$

or, $\mathrm{p}\propto {\mathrm{v}}_{\mathrm{rms}}^2$

but, ${\mathrm{v}}_{\mathrm{rms}}^2\propto \mathrm{T}$

We get,  $\mathrm{p}\propto \mathrm{T}$

7. DERIVATION OF IDEAL GAS EQUATION

All gasses obey Boyle’s law and Charles law at low pressures and high temperatures. The gases which obey the above laws at all pressures and temperatures are known as ideal gases. An ideal gas equation gives the relation between the pressure P, the volume V and the absolute temperature T for a given mass of a gas. Consider a gas of mass one gram having a volume V₁ at pressure P₁ and absolute temperature T₁. Let the pressure, volume of the gas at temperature T., be P₂ and V₂ respectively. We can consider the change of state from P₁V₁ and T₁ to P₂, V₂ and T₂ in two different stages as follows:

Keeping the temperature ‘T₁’ constant, when the pressure of the gas is changed from P to P₂ its volume changes from V₁ to V. According to Boyle’s law P₁V₁= P₂V

$\therefore \mathrm{V}=\frac{{\mathrm{P}}_1{\mathrm{V}}_1}{{\mathrm{P}}_2}$  ……. (1)

Now, keeping the pressure P, constant, if the temperature is changed from T₁ to T₂, the volume of the gas changes from V to V₂. Thus, from Charles law, V $\propto$ T.

$\therefore \mathrm{V}=\frac{{\mathrm{V}}_2{\mathrm{T}}_1}{{ \mathrm{T}}_2}$ ……. (2)

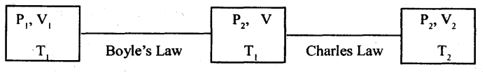
From equations (1) & (2)

$\frac{{\mathrm{P}}_1{\mathrm{V}}_1}{{\mathrm{P}}_2}=\frac{{\mathrm{V}}_2{\mathrm{T}}_1}{{\mathrm{T}}_2}$ 

$\frac{{\mathrm{P}}_1{\mathrm{V}}_1}{{\mathrm{T}}_1}=\frac{{\mathrm{P}}_2{\mathrm{V}}_2}{{\mathrm{T}}_2}$ ……. (3)

or   $\frac{\mathrm{PV}}{\mathrm{T}}$ = Constant = r (say)

PV = rT          ……. (4)

Here, the constant ‘r’ for one gram of a gas is called the specific gas constant. The value of this is different for different gases because one gram of different gases occupy different volumes even at S.T.P. When one gram mole of any gas at N.T.P is considered, the gas constant will be same for all gases and is denoted by ‘R’ because one mole of any gas at S.T.P occupies the same volume of 22.4 litres. Thus, ‘R’ is called the universal gas constant.

Therefore, from equation (4), we can write

PV = RT            ……. (5)

Equation (4) is called ideal gas equation

If the mass of the gas has ‘n’ moles, then the ideal gas equation becomes”

PV = nRT       ……. (6)

Where $\mathrm{n}=\frac{\mathrm{m}}{\mathrm{M}}=\frac{\text{ mass of the gas in grams }}{\text{ Molecular weight in grams }}$

R = Universal gas constant = $8.318 \mathrm{J}–{\mathrm{mole}}^{–1}{ \mathrm{K}}^{–1}$ =  2 Cal / mol K = $0.082\mathrm{Lit}–\mathrm{atm} {\mathrm{mol}}^{–1}{ \mathrm{K}}^{–1}$

8. DEGREES OF FREEDOM (f)

The term degrees of freedom of a system refers to the possible independent motions a system can have.  The independent motions can be translational, rotational or vibrational or any combination of these.

A particle in motion confined to a straight line has only one translational degree of freedom while if the same particle is confined to move in a plane, it will have two translational degrees of freedom.  If the particle is free to move in space, it will have three translational degrees of freedom.

If instead of particle we consider a molecule of a monatomic gas (like He, A, etc.,) which consists of a single atom, the translational motion can take place in any direction in space.  Thus, it can be resolved along three coordinate axes and can have three independent motions and hence 3 degrees of freedom all translational.  A monatomic molecule can also rotate but due to its small moment of inertia rotational kinetic energy is insignificant.  Therefore, it does not possess rotational degrees of freedom.

The molecules of a diatomic gas such as (H₂, O₂, etc., ) are made up of two atoms joined rigidly to one another through a bond.  This cannot only move bodily, but also rotate about one of the three coordinate axes.  However, its moment of inertia about the axis joining the two atoms is negligible compared to that about the other two axes.  Hence, it can have only 2 rotational motions.  Thus, a diatomic molecule has 5 degrees of freedom; 3 translational and 2 rotational.

A nonlinear polyatomic molecule (such as CO₂, H₂O) can rotate about any of three coordinate axes.  Hence, it has 6 degrees of freedom:  one for the PE and one for the KE of vibration.  This is why

1. A diatomic molecule that is free to vibrate (in addition to translation and rotation) will have 7(2+3+2) degrees of freedom (and not 6-a common misconception).

2. An atom in a solid though has no degrees of freedom for translational and rotational motion, due to vibration along 3 axes has 3⨯2 = 6 degrees of freedom (and not 3 like an ideal gas molecule)

9. LAW OF EQUIPARTITION OF ENERGY

According to it the energy of a gas molecule is equally distributed among its various degrees of freedom and each degree of freedom is associated with energy $\frac{1}{2}$kT where k is Boltzmann constant and T temperature of thetas in Kelvin.  According to equipartition theorem, the mean energy (also called average energy, total energy or internal energy) of an ideal gas molecule (i.e. monatomic gas) will be $\frac{3}{2}$kT as it has 3 degrees of freedom.  This result is in agreement with the result of kinetic theory of gases, i.e. the mean energy of ideal gas molecule (which has only translational KE) is $\frac{3}{2}$kT.

In accordance with equipartition of energy, for diatomic and polyatomic gases the average energy per molecule will be $\frac{5}{2}$kT and $\frac{6}{2}$kT respectively (as degrees of freedom for diatomic gas is 5 while for polyatomic gas 6) i.e. at same temperature gases with different degrees of freedom (e.g. He and H₂) will have different average energy(or internal energy) though all have same average translational energy equal to the average energy of an ideal gas molecule, i.e. $\frac{3}{2}$kT

Now if we consider n moles of an ideal (monatomic) gas, the total number of molecules in the gas will be nN_A  (N_A= Avogadro’s number).  Now as energy of one molecule is $\frac{3}{2}$kT, the internal energy of the gas will be

U_mono = nN_A⨯m$\frac{3}{2}$(kT)  = $\frac{3}{2}$nRT [as N_ak = R]

So in general, the internal energy of n moles of a gas in which each molecule has F degrees of freedom will be

U = $\frac{1}{2}$nFRT

e.g., for diatomic gas     F = 5,   so U = $\frac{5}{2}\mathrm{nRT}$

while for polyatomic gas  F = 6 so U = $\frac{6}{2}\mathrm{nRT}$

10. MOLAR HEAT CAPACITY

Specific heat capacity or simply specific heat of a substance is defined as the heat required to raise the temperature of unit mass of that substance through 1^oC (or 1K), i.e.

$\mathrm{c}=\frac{\mathrm{\Delta Q}}{\mathrm{m\Delta T}}$

Now as heat is path dependent, the specific heat will also depend on the conditions of experiment (i.e. the way in which heat is supplied to the body).  In general, experiments are made either at constant volume or at constant pressure.  In case of solids and liquids, due to small thermal expansion, the difference in measured values of specific heats is very small and is usually neglected.  However, in case of gases, specific heat at constant volume c_V is quite different from that at constant pressure c_p.

The specific heat of a gas at constant volume is defined as the quantity of heat required to raise the temperature of unit mass of gas through 1K when its volume is kept constant, i.e.,  

${\mathrm{C}}_{\mathrm{V}}=\frac{\left(\Delta \mathrm{Q}\right)\mathrm{V}}{\mathrm{m}\Delta \mathrm{T}}$

The specific heat of a gas at constant pressure is defined as quantity of heat required to raise the temperature of unit mass of gas through 1K when its pressure is kept constant, i.e.  

${\mathrm{c}}_{\mathrm{P}}=\frac{(\mathrm{\Delta Q}{)}_{\mathrm{P}}}{\mathrm{m\Delta T}}$

If instead of unit mass, 1 mole of gas is considered the specific heats are called molar heats or molar heat capacities and are represented by C_V and C_P respectively, i.e.  

${\mathrm{C}}_{\mathrm{V}}={\mathrm{Mc}}_{\mathrm{V}}=\frac{(\mathrm{\Delta Q}{)}_{\mathrm{V}}}{\mathrm{nQT}} \left[\text{ as }\mathrm{n}=\frac{\mathrm{m}}{\mathrm{M}}\right]$

and   ${\mathrm{C}}_{\mathrm{P}}={\mathrm{Mc}}_{\mathrm{P}}=\frac{(\mathrm{\Delta Q}{)}_{\mathrm{P}}}{\mathrm{\mu \Delta T}} \left[\text{ as }\mathrm{n}=\frac{\mathrm{m}}{\mathrm{M}}\right]$

In case of constant volume the heat supplied to the gas is used only in increasing the temperature, i.e., internal energy of gas, i.e.,

$(\mathrm{\Delta Q}{)}_{\mathrm{V}}=\mathrm{\Delta U}={\mathrm{nC}}_{\mathrm{V}}\mathrm{\Delta T}$  . . . . (1)

While heat supplied to the gas at constant pressure, by definition of C_P is

$(\mathrm{\Delta Q}{)}_{\mathrm{P}}={\mathrm{nC}}_{\mathrm{P}}\mathrm{\Delta T}$. . . .      (2)

This heat is used in two ways, viz;

(a) in increasing the temperature of the gas by

ΔT = ΔU

(b) in doing work due to expansion at constant pressure = ΔW

so   $(\mathrm{\Delta Q}{)}_{\mathrm{P}}=\mathrm{\Delta U}+\mathrm{\Delta W}$    . . . . (3)

substituting the values of (ΔQ)_P and ΔU from equations (1) and (2) in (3), we have

${\mathrm{nC}}_{\mathrm{P}}\Delta \mathrm{T}={\mathrm{nC}}_{\mathrm{V}}\Delta \mathrm{T}+\mathrm{P}\Delta \mathrm{V}$ ($\therefore$ at constant, P, ΔW = PΔV)

or  ${\mathrm{C}}_{\mathrm{P}}–{\mathrm{C}}_{\mathrm{V}}=\frac{\mathrm{P\Delta V}}{\mathrm{n\Delta T}}$    . . . .      (4)

Now as for ideal gas PV = nRT, at constant pressure

PΔV = nRΔT

$\therefore C_P–C_V=R$    . . . .      (5)

This relation is called Mayer’s relation and shows that C_P > C_V, i.e. molar thermal capacity (or specific heat) at constant pressure is greater than that at constant volume.

Now as for a gas having F degrees of freedom,

$\mathrm{U}=\frac{1}{2}\mathrm{nRFT}$,

i.e. ${\mathrm{C}}_{\mathrm{V}}=\frac{(\mathrm{\Delta Q}{)}_{\mathrm{V}}}{\mathrm{n\Delta T}}=\frac{\mathrm{\Delta U}}{\mathrm{n\Delta T}}=\frac{1}{2}\mathrm{RF}$

so   ${\mathrm{C}}_{\mathrm{V}}=\frac{(\mathrm{\Delta Q}{)}_{\mathrm{V}}}{\mathrm{n\Delta T}}=\frac{\mathrm{\Delta U}}{\mathrm{n\Delta T}}=\frac{1}{2}\mathrm{RF}$

${\mathrm{C}}_{\mathrm{P}}={\mathrm{C}}_{\mathrm{V}}+\mathrm{R}=\left(1+\frac{\mathrm{F}}{2}\right)\mathrm{R}$

and $\mathrm{\gamma }=\frac{{\mathrm{C}}_{\mathrm{P}}}{{\mathrm{C}}_{\mathrm{V}}}=1+\frac{2}{\mathrm{F}}$

so for monatomic gases, like He, Li, as F = 3,

${\mathrm{C}}_{\mathrm{V}}=\frac{3}{2}\mathrm{R}; {\mathrm{C}}_{\mathrm{P}}=\frac{5}{3}\mathrm{R}\text{ and }\gamma =\frac{5}{3}=1.66$

and and for diatomic gases like H₂, O₂, as F=5,

${\mathrm{C}}_{\mathrm{V}}=\frac{5}{2}\mathrm{R}; {\mathrm{C}}_{\mathrm{P}}=\frac{7}{2}\mathrm{R} \text{and }\gamma =\frac{7}{5}=1.4$

while for polyatomic gases like NH₃, CO_­2, as F = 6,

${\mathrm{C}}_{\mathrm{V}}=3\mathrm{R}; {\mathrm{C}}_{\mathrm{P}}=4\mathrm{R}\text{ and }\gamma =\frac{4}{3}=1.33$

From these expressions it is clear that γ varies with atomicity (number of atoms in a molecule) and with increase in atomicity it decreases.  So a knowledge of γ helps in determining the atomicity of gases and hence, in understanding their molecular constitution.

SOLVED EXAMPLES  

1. Calculate the root mean square speed of smoke particles of mass 5⨯10^–17 kg in their Brownian motion in air at NTP (k= 1.38⨯10^–23 J/K).

Sol. According to the kinetic theory of gases,

${\mathrm{v}}_{\mathrm{rms}}=\sqrt{\frac{3\mathrm{RT}}{\mathrm{M}}}=\sqrt{\frac{3\mathrm{kT}}{\mathrm{m}}}$ [as R = N_Ak and M = N_Am]

Here T = 273K; m = 5⨯10^–17 kg

And  k = 1.38⨯10^–23 J/K

So ${\mathrm{v}}_{\mathrm{rms}}=\sqrt{\frac{3\times 1.38\times {10}^{–23}\times 273}{5\times {10}^{–17}}}$

= $1.5\times {10}^{–2} \mathrm{m}/\mathrm{s}=1.5$ cm/s

2. Two vessels having equal volume contain molecular hydrogen at one atmosphere and helium at two atmospheres respectively.  What is the ratio of rms speed of hydrogen molecule to that of helium molecule if both the samples are at same temperature?

Sol. According to kinetic theory of gases,

${\mathrm{v}}_{\mathrm{rms}}=\sqrt{\frac{3\mathrm{RT}}{\mathrm{M}}}; \frac{{\mathrm{v}}_{\mathrm{H}}}{{\mathrm{V}}_{\mathrm{He}}}=\sqrt{\frac{M_{\mathrm{He}}}{{\mathrm{M}}_{\mathrm{H}}}}$

i.e. ${\mathrm{v}}_{\mathrm{H}}=\sqrt{\left(\frac{4}{2}\right)}{\mathrm{V}}_{\mathrm{He}}=\sqrt{2}\left({\mathrm{v}}_{\mathrm{He}}\right)$

3. At what temperature will the rms speed of oxygen molecule will be sufficient for escaping from the earth? (v_e = 11.2 km/s, m = 2.7610^–26 kg and k = 1.38⨯10^–23 J/K)

Sol. If the temperature is T, according to kinetic theory of gases,

Translational KE = $\left(\frac{3}{2}\right)\mathrm{kT}$

The oxygen molecule will escape from earth if,  

$\frac{3}{2}\mathrm{kT}>\frac{1}{2}{\mathrm{mv}}_{\mathrm{e}}^2$

i.e. $\mathrm{T}>\frac{{\mathrm{mv}}_{\mathrm{e}}^2}{3\mathrm{k}}\text{ or }\mathrm{T}>\frac{2.76\times {10}^{–26}\times {\left(11.2\times {10}^3\right)}^2}{3\times 1.38\times {10}^{–23}}$

i.e.    $\mathrm{T}>8.36\times {10}^4 \mathrm{K}$

4. At what temperature does the average translational kinetic energy of a molecule in a gas become equal to the KE of an electron accelerated from rest through a potential difference of 1 volt? (k = 1.38⨯10^-23 J/k).

Sol. When an electron is accelerated by 1 volt, its kinetic energy will be 1eV.  Now as according to kinetic theory of gases KE = $\left(\frac{3}{2}\right)\mathrm{kT}$,

$\frac{3}{2}\mathrm{kT}=1\mathrm{eV}=1.6\times {10}^{–19} \mathrm{J}$

i.e $T=\frac{2}{3}\times \frac{1.6\times {10}^{–19}}{1.38\times {10}^{–23}}=7730 \mathrm{K}$

5. Dust particles in the suspended state in a monatomic gas are in thermal equilibrium with the gas. If the temperature of the gas is 300K, find the mean KE of translation of dust particles.  If the mass of a certain particle is 10^-17 kg, calculate its rms speed.  (k=1.38⨯10^-23 J/K).

Sol. According to kinetic theory of gases, KE = $\frac{3}{2}$kT, so for a particle in thermal equilibrium T_P = T_G= T.

So = 6.21⨯10^–21 J

Now if m is the mass of particle and v_rms, rms speed,

$\mathrm{KE}=\frac{1}{2}{\mathrm{mv}}_{\mathrm{rms}}^2 \text{ i.e., }{\mathrm{v}}_{\mathrm{rms}}=\sqrt{\frac{2\mathrm{KE}}{\mathrm{m}}}$

$\therefore {\mathrm{v}}_{\mathrm{rms}}=\sqrt{\frac{2\times 6.21\times {10}^{–21}}{{10}^{–17}}}$ = $3.524\times {10}^{–2} \mathrm{m}/\mathrm{s}\simeq 3.5 \mathrm{cm}/\mathrm{s}$

6. The temperature of a gas is -68^oC. To what temperature should it be heated so that (a) the average translational KE of the molecules be doubled?  (b) the root mean square velocity of the molecules be doubled?

Sol. (a) According to kinetic theory of gases, average translational KE of a molecule is given by

$\mathrm{E}=\frac{3}{2}\mathrm{kT}\text{ so that }\frac{{\mathrm{E}}_2}{{\mathrm{E}}_1}=\frac{{\mathrm{T}}_2}{{\mathrm{T}}_1}$

According to the given problem

So,  ${\mathrm{T}}_2=2{\mathrm{T}}_1=2[273+(–68\left)\right]=410 \mathrm{K}\left(=137°\mathrm{C}\right)$

b) According to kinetic theory of gases, rms speed of gas molecules is given by

${\mathrm{v}}_{\mathrm{rms}}=\sqrt{\frac{3\mathrm{RT}}{\mathrm{M}}} \text{so that }\frac{{\left({\mathrm{v}}_{\mathrm{rms}}\right)}_2}{{\left({\mathrm{v}}_{\mathrm{rms}}\right)}_1}=\sqrt{\frac{{\mathrm{T}}_2}{{ \mathrm{T}}_1}}$

According to the given problem ${\left({\mathrm{v}}_{\mathrm{rms}}\right)}_2=2{\left({\mathrm{v}}_{\mathrm{rms}}\right)}_1$

So  ${\mathrm{T}}_2=4{\mathrm{T}}_1=4(273+(–68\left)\right)=820 \mathrm{K}\left(=547°\mathrm{C}\right)$

7. One mole of a monoatomic gas is mixed with 3 moles of a diatomic gas. What is the molecular specific heat of the mixture at constant volume?  (R=8.31 J/mol K).

Sol. For monoatomic gas C_V = $\frac{3}{2}$R while diatomic, $\frac{5}{2}\mathrm{R}$, so by conservation of energy.    

${\mathrm{C}}_{\mathrm{V}}=\frac{{\mathrm{n}}_1{\left({\mathrm{C}}_{\mathrm{V}}\right)}_1+{\mathrm{n}}_2{\left({\mathrm{C}}_{\mathrm{V}}\right)}_2}{{\mathrm{n}}_1+{\mathrm{n}}_2}=\frac{1\times \frac{3}{2}\mathrm{R}+3\times \frac{5}{2}\mathrm{R}}{1+3}$

i.e.  ${\mathrm{C}}_{\mathrm{V}}=\frac{9}{4}\mathrm{R}=\frac{9}{4}\times 8.31=18.7$ J/mol K  

8. A gaseous mixture enclosed in a vessel consists of one gram mole of a gas A with $\gamma =\frac{5}{3}$ and another B with $\gamma =\frac{7}{5}$ at a temperature T.  The gases A and B do not react with ach other and assumed to be ideal.  Find the number of gram moles of the gas B.  If γ for the gaseous mixture is $\frac{19}{13}$.

Sol. As for ideal gas ${\mathrm{C}}_{\mathrm{P}}–{\mathrm{C}}_{\mathrm{P}}=\mathrm{R}\text{ and }\mathrm{\gamma }=\frac{{\mathrm{C}}_{\mathrm{p}}}{{\mathrm{C}}_{\mathrm{v}}}$

$\gamma –1=\frac{\mathrm{R}}{{\mathrm{C}}_{\mathrm{V}}}\text{ or }{\mathrm{C}}_{\mathrm{V}}=\frac{\mathrm{R}}{(\gamma –1)}$

$\therefore {\mathrm{C}}_{\mathrm{v}1}=\frac{\mathrm{R}}{\frac{5}{3}–1}=\frac{3}{2}\mathrm{R}; {\mathrm{C}}_{\mathrm{V}2}=\frac{\mathrm{R}}{\frac{7}{2}–1}=\frac{5}{2}\mathrm{R}$

and ${\mathrm{C}}_{\mathrm{V}\text{ mix }}=\frac{\mathrm{R}}{\frac{19}{13}–1}=\frac{13}{6}\mathrm{R}$

Now from conservation of energy, i.e., ΔU = ΔU₁ + ΔU₂,

i.e., ${\mathrm{C}}_{\mathrm{Vmix}}=\frac{{\mathrm{n}}_1{\mathrm{C}}_{\mathrm{V}1}+{\mathrm{n}}_2{\mathrm{C}}_{\mathrm{V}2}}{{\mathrm{n}}_1+{\mathrm{n}}_2}$

we have $\frac{13}{6}\mathrm{R}=\frac{1\times \frac{3}{2}\mathrm{R}+\mathrm{n}\times \frac{5}{2}\mathrm{R}}{1+\mathrm{n}}=\frac{\left(35\mathrm{n}\right)\mathrm{R}}{2(1+\mathrm{n})}$

or 13 + 13n = 9 + 15n, i..e. n = 2 gram mole